AI tutor
Full solution
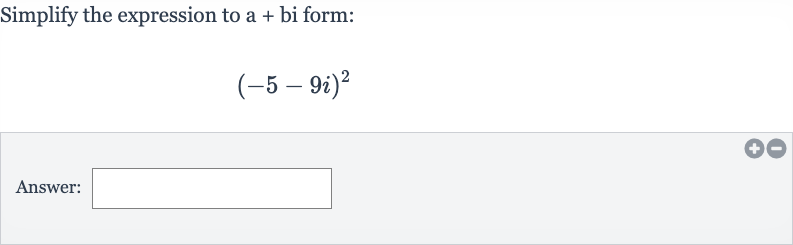
Q. Simplify the expression to a + bi form:Answer:
- Apply Formula: To simplify the expression , we need to square the complex number . This involves using the formula .
- Square Real Part: First, we square the real part: .
- Square Imaginary Part: Next, we square the imaginary part: . Remembering that , we get .
- Calculate Middle Term: Now, we calculate the middle term, which is times the product of the real part and the imaginary part: .
- Combine Terms: We combine all the terms to get the simplified expression: .