Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

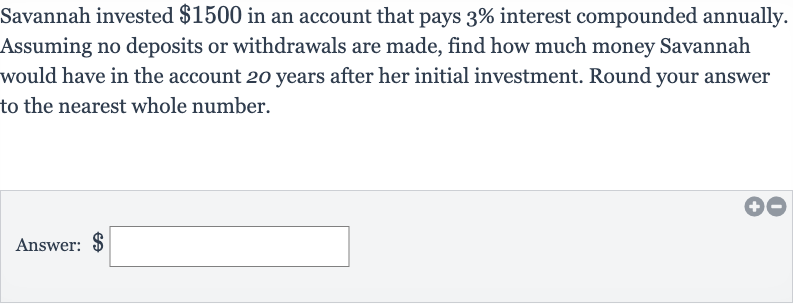
Savannah invested
$1500 in an account that pays
3% interest compounded annually. Assuming no deposits or withdrawals are made, find how much money Savannah would have in the account 20 years after her initial investment. Round your answer to the nearest whole number.
Answer: $ □

Savannah invested $\$ 1500$ in an account that pays $3 \%$ interest compounded annually. Assuming no deposits or withdrawals are made, find how much money Savannah would have in the account $20$ years after her initial investment. Round your answer to the nearest whole number. $\newline$ Answer: $ \(\square\)

View step-by-step help

View step-by-step help

Exponential growth and decay: word problems

Full solution

Q. Savannah invested

\$ 1500

in an account that pays

3 \%

interest compounded annually. Assuming no deposits or withdrawals are made, find how much money Savannah would have in the account

20

years after her initial investment. Round your answer to the nearest whole number.

\newline

Answer: $ \(\square\)

Identify Variables: Identify the principal amount $P$ , the annual interest rate $r$ , the number of times the interest is compounded per year $n$ , and the number of years the money is invested $t$ . $P = \$1500$ , $r = 3\%$ or $0.03$ , $n = 1$ (since it's compounded annually), $t = 20$ years.
Compound Interest Formula: Use the compound interest formula $A = P(1 + r/n)^{(nt)}$ to find the amount of money $A$ that Savannah will have in the account after $t$ years. $\newline$ Substitute the known values into the formula: $A = 1500(1 + 0.03/1)^{(1*20)}$ .
Simplify and Calculate Exponent: Simplify the expression inside the parentheses and then calculate the exponent. $\newline$ $A = 1500(1 + 0.03)^{20} = 1500(1.03)^{20}$ .
Calculate Value: Calculate the value of $(1.03)^{20}$ using a calculator. $\newline$ $(1.03)^{20} \approx 1.80611$ .
Multiply Principal Amount: Multiply the principal amount by the result from Step $4$ to find the total amount in the account after $20$ years. $\newline$ $A = 1500 \times 1.80611 \approx 2709.165$ .
Round to Nearest Dollar: Round the result to the nearest whole number as the problem asks for the answer in whole dollars. $\newline$ $A \approx \$2710$ .

More problems from Exponential growth and decay: word problems

Question

Use the following function rule to find

f(2)

\newline

f(x) = 11 \cdot (9)^x + 8

\newline

f(2) =

Posted 5 months ago

Question

Raymond works for a pharmaceutical company that is testing the effectiveness of a new medication. He gave one of the study participants

400

milligrams of the medication, and he knows the amount remaining in the participant's body will decrease by a factor of

1/3

\newline

Write an exponential equation in the form

y = a(b)^x

that can model the amount of medication,

y

, remaining in the participant's body after

x

\newline

Use whole numbers, decimals, or simplified fractions for the values of

a

b

\newline

y =

\newline

Posted 8 months ago

Question

100

bacteria doubles in size every

60

hours. What will the population be

180

?

Posted 4 months ago

Question

Charlotte opened a savings account and deposited

\$800.00

as principal. The account earns

8\%

interest, compounded annually. What is the balance after

10

\newline

Use the formula

A = P(1 + \frac{r}{n})^{nt}

A

is the balance (final amount),

P

is the principal (starting amount),

r

is the interest rate expressed as a decimal,

n

is the number of times per year that the interest is compounded, and

t

is the time in years.

\newline

Round your answer to the nearest cent.

Posted 4 months ago

Question

Bob rented a car for a flat fee of `$150` and an additional `$10` per day. Write a linear function that represents the total cost,

f(x)

x

Posted 10 months ago

Question

Sally purchased a laptop for `$800` and has to pay a service fee of `$20` for each day until it is delivered. Write a linear function to represent the total cost,

h(x)

x

Posted 10 months ago

Question

\$1,000

in a savings account with a daily interest rate of

0.1\%

. Write an exponential function to represent the total amount,

s(x)

, in his account after

x

Posted 9 months ago

Question

A=2450+740 s

\newline

The total amount,

A

, in thousands of dollars, of scholarship money given out by a college throughout its history at a time of

s

semesters after the summer of the year

2000

is given by the equation. What was the total amount of scholarship money, in thousands of dollars, given out by the college up to the summer of the year

2000

Posted 5 months ago

Question

T=235+980 d

\newline

T

, in newton-meters, on a diving board with a

100

kilogram weight placed

d

meters from the diving board's fulcrum is given by the equation. How much torque in newton-meters is on the diving board when the weight is placed at the fulcrum?

Posted 8 months ago

Question

R=42-0.7 t

\newline

Quinn returned home one summer's day to find it extremely hot. He turned the air conditioner on, and the room's temperature began decreasing at a constant rate. The given equation shown gives the room's temperature,

R

, in degrees Celsius,

t

minutes after Quinn turned on the air conditioner. What was the room's temperature, in degrees Celsius, when Quinn returned home?

Posted 7 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant