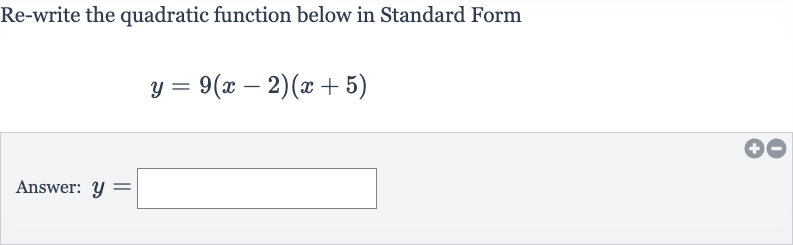
Re-write the quadratic function below in Standard Form y=9(x-2)(x+5) Answer: y=

Question

Re-write the quadratic function below in Standard Form  y=9(x-2)(x+5) Answer:  y=

Bytelearn · Accepted Answer

Expand Binomials: To rewrite the quadratic function in standard form, we need to expand the product of the binomials in the function y = 9(x - 2)(x + 5). First, we'll apply the distributive property (also known as the FOIL method for binomials) to multiply the two binomials (x - 2) and (x + 5). Let's multiply the first terms, the outer terms, the inner terms, and the last terms of the binomials. First terms: x * x = x^2 Outer terms: x * 5 = 5x Inner terms: -2 * x = -2x Last terms: -2 * 5 = -10 Now, we combine these results to get the expanded form. x^2 + 5x - 2x - 10 Combine like terms (5x and -2x) to simplify the expression. x^2 + (5x - 2x) - 10 x^2 + 3x - 10Combine Like Terms: Now that we have the expanded form of the binomials, we need to multiply this result by the coefficient 9 to get the quadratic function in standard form. Multiply each term in the expression x^2 + 3x - 10 by 9. 9 * x^2 = 9x^2 9 * 3x = 27x 9 * (-10) = -90 Combine these results to get the final standard form of the quadratic function. 9x^2 + 27x - 90

Re-write the quadratic function below in Standard Form $\newline$ $y=9(x-2)(x+5)$ $\newline$ Answer: $y=$

Full solution

More problems from Multiply two binomials

Related topics

Re-write the quadratic function below in Standard Form\newliney=9(x−2)(x+5) y=9(x-2)(x+5) y=9(x−2)(x+5)\newlineAnswer: y= y= y=

Re-write the quadratic function below in Standard Form $\newline$ $y=9(x-2)(x+5)$ $\newline$ Answer: $y=$