AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
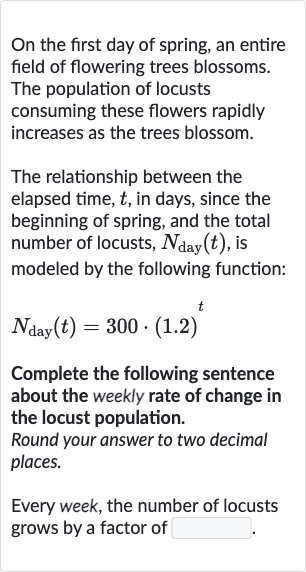
On the first day of spring, an entire field of flowering trees blossoms. The population of locusts consuming these flowers rapidly increases as the trees blossom.The relationship between the elapsed time, , in days, since the beginning of spring, and the total number of locusts, , is modeled by the following function:Complete the following sentence about the weekly rate of change in the locust population.Round your answer to two decimal places.Every week, the number of locusts grows by a factor of
Full solution
Q. On the first day of spring, an entire field of flowering trees blossoms. The population of locusts consuming these flowers rapidly increases as the trees blossom.The relationship between the elapsed time, , in days, since the beginning of spring, and the total number of locusts, , is modeled by the following function:Complete the following sentence about the weekly rate of change in the locust population.Round your answer to two decimal places.Every week, the number of locusts grows by a factor of
- Substitute with : To find the weekly rate of change, we need to calculate the factor by which the number of locusts grows in one week. Since one week is equivalent to days, we will substitute with in the given function .
- Calculate : Now, let's calculate the value of to find out the number of locusts at the end of one week.
- Calculate : Perform the calculation for .
- Multiply by : Now, multiply the result by to get the total number of locusts after one week.
- Find factor of growth: However, we are interested in the factor of growth, not the actual number of locusts. The factor of growth is simply since the initial population is multiplied by this factor after one week.
- Round to two decimal places: Round the factor of growth to two decimal places.The weekly growth factor is approximately .
