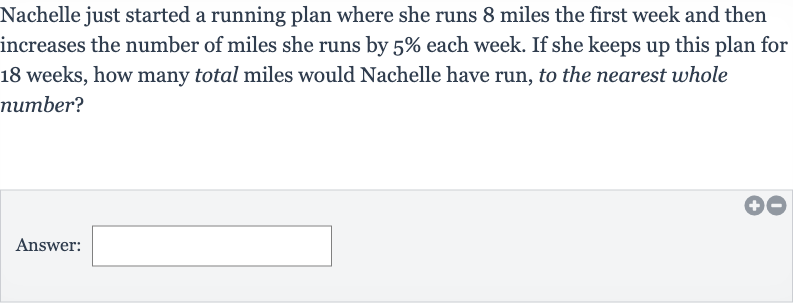
Nachelle just started a running plan where she runs 8 miles the first week and then increases the number of miles she runs by 5% each week. If she keeps up this plan for 18 weeks, how many total miles would Nachelle have run, to the nearest whole number? Answer:

Question

Nachelle just started a running plan where she runs 8 miles the first week and then increases the number of miles she runs by  5% each week. If she keeps up this plan for 18 weeks, how many total miles would Nachelle have run, to the nearest whole number? Answer:

Bytelearn · Accepted Answer

Identify: Identify the initial amount of miles and the rate of increase. Nachelle starts with 8 miles and increases her running distance by 5% each week. Initial miles (a) = 8 Rate of increase (r) = 5% or 0.05Determine formula: Determine the formula for the total distance run after n weeks. The total distance run is the sum of a geometric series where each term is 5% greater than the previous term. The formula for the sum of the first n terms of a geometric series is S_n = a(1 - r^n) / (1 - r), where r is not equal to 1. In this case, r = 1.05 (since it's a 5% increase, or 1 + 0.05).Calculate total distance: Calculate the total distance run after 18 weeks. Substitute the values into the formula: S_18 = 8(1 - 1.05^18) / (1 - 1.05)Evaluate formula: Evaluate the formula. First, calculate 1.05^18: 1.05^18 ≈ 2.39656Continue evaluating: Continue evaluating the formula. Now, substitute 2.39656 into the formula: S_18 = 8(1 - 2.39656) / (1 - 1.05)Simplify expression: Simplify the expression. Calculate the numerator: 8(1 - 2.39656) = 8(-1.39656) ≈ -11.17248Calculate denominator: Calculate the denominator: 1 - 1.05 = -0.05Divide to find S_18: Divide the numerator by the denominator to find S_18. S_18 = -11.17248 / -0.05 ≈ 223.4496Round total distance: Round the total distance to the nearest whole number. S_18 ≈ 223 miles

Nachelle just started a running plan where she runs $8$ miles the first week and then increases the number of miles she runs by $5 \%$ each week. If she keeps up this plan for $18$ weeks, how many total miles would Nachelle have run, to the nearest whole number? $\newline$ Answer:

Full solution

More problems from Exponential growth and decay: word problems

Related topics

Nachelle just started a running plan where she runs 888 miles the first week and then increases the number of miles she runs by 5% 5 \% 5% each week. If she keeps up this plan for 181818 weeks, how many total miles would Nachelle have run, to the nearest whole number?\newlineAnswer:

Nachelle just started a running plan where she runs $8$ miles the first week and then increases the number of miles she runs by $5 \%$ each week. If she keeps up this plan for $18$ weeks, how many total miles would Nachelle have run, to the nearest whole number? $\newline$ Answer: