Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

log_(3)9=

$\log _{3} 9=$

View step-by-step help

View step-by-step help

Add, subtract, multiply, and divide integers

Full solution

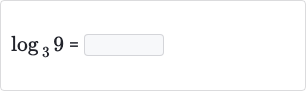
Q.

\log _{3} 9=

Identify base and number: Identify the base and the number for which we are finding the logarithm. $\newline$ The base is $3$ and the number is $9$ .
Recall logarithm definition: Recall the definition of a logarithm. $\newline$ The logarithm $\log_{\text{base}}(b)(a)$ is the exponent to which the base $b$ must be raised to obtain the number $a$ .
Determine exponent: Determine the exponent that makes the base equal to the number. $\newline$ We need to find the exponent $x$ such that $3^x = 9$ .
Solve equation: Solve the equation $3^x = 9$ . $\newline$ Since $9$ is $3$ squared ( $3^2$ ), we can see that $x = 2$ .
Conclude logarithm value: Conclude the value of the logarithm. $\newline$ Therefore, $\log_{3}9 = 2$ .

More problems from Add, subtract, multiply, and divide integers

Question

Write an expression to represent:

7

minus the product of

3

x

Posted 3 months ago

Question

Write an expression to represent:

9

minus the quotient of

2

x

Posted 3 months ago

Question

Write an expression to represent:

\newline

2

times the sum of

5

x

Posted 3 months ago

Question

\newline

\frac{2}{7} \div \frac{8}{3}=

Posted 3 months ago

Question

\newline

\frac{2}{5} \div \frac{8}{5}=

Posted 3 months ago

Question

\newline

\frac{8}{5} \div \frac{7}{8}=

Posted 3 months ago

Question

\newline

\frac{1}{4} \div \frac{8}{3}=

Posted 3 months ago

Question

\newline

\frac{2}{7} \div \frac{4}{9}=

Posted 3 months ago

Question

\newline

\frac{2}{5} \div \frac{1}{7}=

Posted 3 months ago

Question

\newline

\frac{4}{7} \div \frac{7}{2}=

Posted 3 months ago