Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

log_(3)(1)/(27)=

log⁡3127= \log _{3} \frac{1}{27}=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Evaluate logarithms using propertiesright chevron icon

Full solution

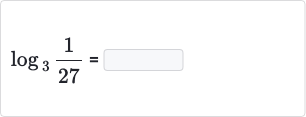
Q. log⁡3127= \log _{3} \frac{1}{27}=
  1. Recognize Power of 33: We are asked to find the value of the logarithm of 127\frac{1}{27} with base 33. The first step is to recognize that 2727 is a power of 33. \newline27=3327 = 3^3
  2. Rewrite as Negative Power: Now, we can rewrite 127\frac{1}{27} as 33 raised to a negative power because 127\frac{1}{27} is the reciprocal of 2727. \newline127=133=3−3\frac{1}{27} = \frac{1}{3^3} = 3^{-3}
  3. Apply Logarithm Definition: Next, we can apply the definition of a logarithm. The logarithm log⁡3(3−3)\log_{3}(3^{-3}) asks for the exponent that the base 33 must be raised to in order to yield 3−33^{-3}.\newlinelog⁡3(3−3)=−3\log_{3}(3^{-3}) = -3
  4. Final Logarithm Value: Since the base 33 raised to the power of −3-3 gives us 127\frac{1}{27}, the logarithm log⁡3(127)\log_{3}(\frac{1}{27}) is equal to −3-3.

More problems from Evaluate logarithms using properties

Question
Write the exponential equation in logarithmic form.\newline93=7299^3 = 729\newlinelog⁡□729=3\log_\square 729 = 3
Get tutor helpright-arrow

Posted 2 months ago

Question
Write the exponential equation in logarithmic form.\newlinee4≈54.598e^4 \approx 54.598\newlineln⁡____≈4\ln\_\_\_\_ \approx 4
Get tutor helpright-arrow

Posted 2 months ago

Question
Write the logarithmic equation in exponential form.\newlinelog⁡10100=2\log_{10}100 = 2\newline102=‾10^2 = \underline{\hspace{2em}}
Get tutor helpright-arrow

Posted 2 months ago

Question
Evaluate. Write your answer as a whole number, proper fraction, or improper fraction in simplest form.\newlineln⁡(e)10=\frac{\ln (e)}{10} = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Rewrite as a quotient of two common logarithms. Write your answer in simplest form.\newlinelog⁡333=\log_3 33 = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Evaluate. Round your answer to the nearest thousandth.\newlinelog⁡550=\log_{5}50 = ____
Get tutor helpright-arrow

Posted 2 months ago

Question
Which property of logarithms does this equation demonstrate? \newlinelog⁡33+log⁡36=log⁡318\log_3 3 + \log_3 6 = \log_3 18\newlineChoices:\newline(A) Product Property\text{Product Property}\newline(B) Power Property\text{Power Property}\newline(C) Quotient Property\text{Quotient Property}
Get tutor helpright-arrow

Posted 2 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of common logarithms or multiples of common logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡(uv)\log(uv)\newline_____
Get tutor helpright-arrow

Posted 2 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of common logarithms or multiples of common logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡v7\log v^7 \newline______
Get tutor helpright-arrow

Posted 2 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of base-66 logarithms or multiples of base-66 logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡6w6\log_6 w^6\newline______
Get tutor helpright-arrow

Posted 2 months ago

View all (27)