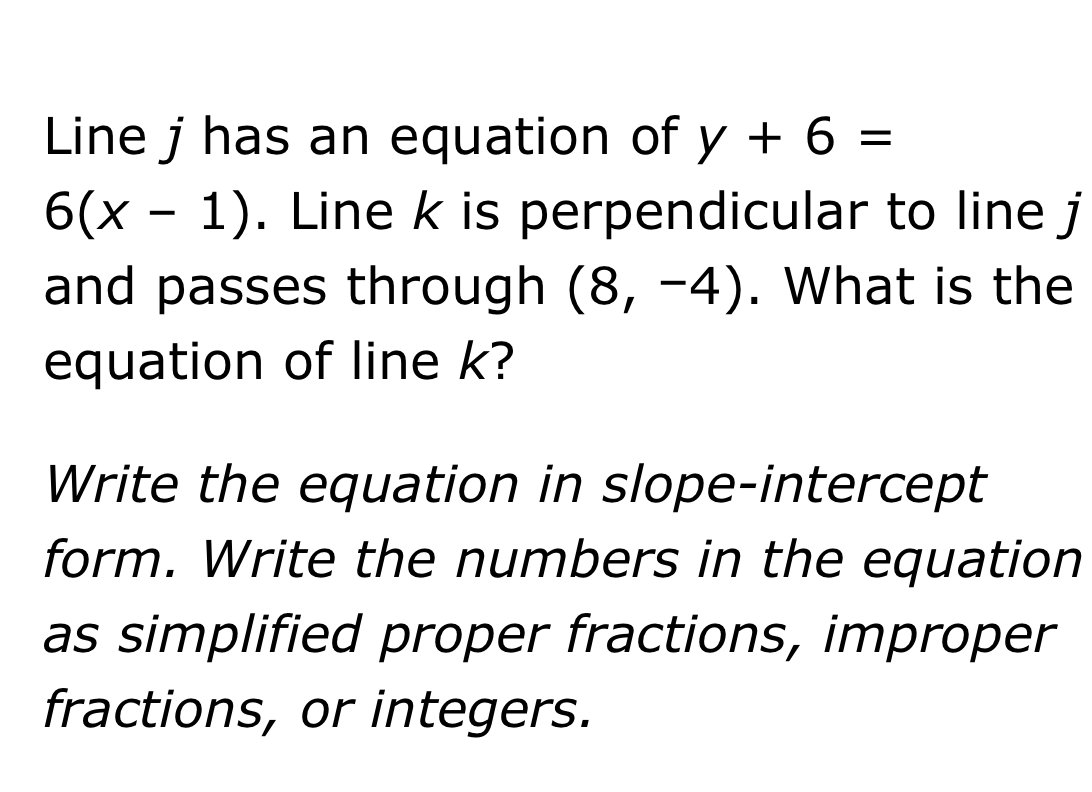
Line j has an equation of y+6= 6(x-1). Line k is perpendicular to line j and passes through (8,-4). What is the equation of line k ? Write the equation in slope-intercept form. Write the numbers in the equation as simplified proper fractions, improper fractions, or integers.

Question

Line  j has an equation of  y+6=  6(x-1). Line  k is perpendicular to line  j and passes through  (8,-4). What is the equation of line  k ? Write the equation in slope-intercept form. Write the numbers in the equation as simplified proper fractions, improper fractions, or integers.

Bytelearn · Accepted Answer

Find Slope of Line j: First, we need to find the slope of line j by putting its equation into slope-intercept form (y = mx + b). y + 6 = 6(x - 1) y = 6x - 6 - 6 y = 6x - 12 The slope (m) of line j is 6.Determine Slope of Line k: Since line k is perpendicular to line j, its slope will be the negative reciprocal of the slope of line j. The negative reciprocal of 6 is -1/6. So, the slope (m) of line k is -1/6.Use Point-Slope Form: Now we have the slope of line k and a point (8, -4) through which it passes. We can use the point-slope form to find the equation of line k. The point-slope form is y - y1 = m(x - x1), where m is the slope and (x1, y1) is the point.Convert to Slope-Intercept Form: Plugging the slope and the point into the point-slope form, we get: y - (-4) = -1/6(x - 8) y + 4 = -1/6x + 8/6 y + 4 = -1/6x + 4/3To get the equation into slope-intercept form, we need to isolate y: y = -1/6x + 4/3 - 4 y = -1/6x + 4/3 - 12/3 y = -1/6x - 8/3

Full solution

More problems from Write an equation for a parallel or perpendicular line

Related topics