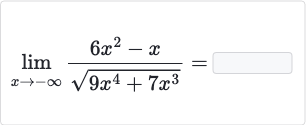Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

lim_(x rarr-oo)(6x^(2)-x)/(sqrt(9x^(4)+7x^(3)))=

$\lim _{x \rightarrow-\infty} \frac{6 x^{2}-x}{\sqrt{9 x^{4}+7 x^{3}}}=$

View step-by-step help

View step-by-step help

Relationship between squares and square roots

Full solution

Q.

\lim _{x \rightarrow-\infty} \frac{6 x^{2}-x}{\sqrt{9 x^{4}+7 x^{3}}}=

Simplify expression by dividing: To find the limit of the given function as $x$ approaches negative infinity, we can simplify the expression by dividing the numerator and the denominator by the highest power of $x$ in the denominator, which is $x^2$ .
Remove terms as $x$ approaches negative infinity: Divide both the numerator and the denominator by $x^2$ :
$\lim_{x \to -\infty} \left[\frac{6x^2 - x}{x^2}\right] / \left[\frac{\sqrt{9x^4 + 7x^3}}{x^2}\right]$
= $\lim_{x \to -\infty} \left[\frac{6 - \frac{1}{x}}{\sqrt{9 + \frac{7}{x}}}\right]$
Simplify expression further: As $x$ approaches negative infinity, the terms $\frac{1}{x}$ and $\frac{7}{x}$ will approach $0$ . Therefore, we can simplify the expression further by removing these terms: $\newline$ $\lim_{x \to -\infty} \frac{6 - 0}{\sqrt{9 + 0}}$ $\newline$ $= \lim_{x \to -\infty} \frac{6}{\sqrt{9}}$
Simplify constant expression: Now we can simplify the constant expression: $6 / \sqrt{9} = 6 / 3 = 2$

More problems from Relationship between squares and square roots

Question

\sqrt{49}

?

\newline

Posted 1 month ago

Question

Complete the following statement. Use the integers that are closest to the number in the middle.

\newline

<

\sqrt{40}

<

Posted 1 month ago

Question

-\sqrt{64}

\newline

Posted 2 months ago

Question

\sqrt[3]{27}

Posted 1 month ago

Question

Which two integers is

-\sqrt{48}

\newline

\newline

[A]-6 \text{ and } -5

\newline

[B]5 \text{ and } 6

\newline

[C]-7 \text{ and } -6

\newline

[D]6 \text{ and } 7

Posted 6 days ago

Question

What is the cube root of

-64

\newline

Posted 2 months ago

Question

Which two integers is

\sqrt[3]{22}

\newline

\newline

[1 \text{ and } 2]

\newline

[15 \text{ and } 16]

\newline

[16 \text{ and } 17]

\newline

[2 \text{ and } 3]

Posted 2 months ago

Question

\newline

(\sqrt{25})^2 = \_\_\_\_\_

Posted 1 month ago

Question

Look at this equation:

\newline

c^3 = 27

\newline

\newline

c

, the cube root of

27

\newline

c =

\newline

Posted 2 months ago

Question

Write the sentence as an equation.

\newline

172

is equal to the quotient of

x

4

\newline

/

) if you want to use a division sign.

\newline

Posted 1 month ago