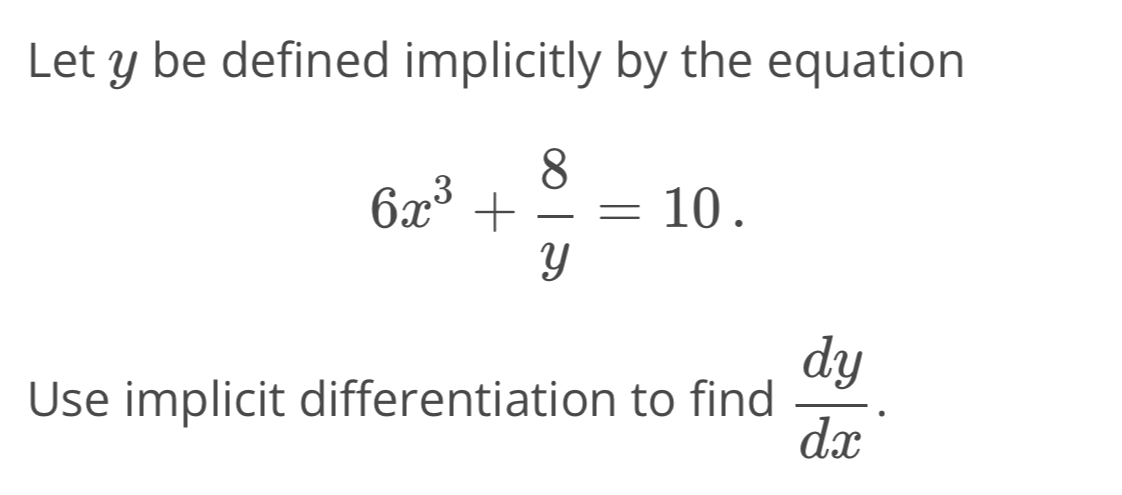Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Let
y be defined implicitly by the equation

6x^(3)+(8)/(y)=10". "
Use implicit differentiation to find
(dy)/(dx).

Let $y$ be defined implicitly by the equation $\newline$ $6 x^{3}+\frac{8}{y}=10 \text {. }$ $\newline$ Use implicit differentiation to find $\frac{d y}{d x}$ .

View step-by-step help

View step-by-step help

Complex conjugate theorem

Full solution

Q. Let

y

be defined implicitly by the equation

\newline

6 x^{3}+\frac{8}{y}=10 \text {. }

\newline

Use implicit differentiation to find

\frac{d y}{d x}

.

Differentiate $6x^3$ : To find the derivative $\frac{dy}{dx}$ using implicit differentiation, we need to differentiate both sides of the equation with respect to $x$ . The equation is $6x^3 + \frac{8}{y} = 10$ .
Differentiate $\frac{8}{y}$ : Differentiate $6x^3$ with respect to $x$ . The derivative of $6x^3$ with respect to $x$ is $18x^2$ .
Differentiate $10$ : Differentiate $\frac{8}{y}$ with respect to $x$ . Since $y$ is a function of $x$ , we need to use the chain rule. The derivative of $\frac{8}{y}$ with respect to $x$ is $-\frac{8}{y^2} * \frac{dy}{dx}$ .
Combine derivatives: Differentiate $10$ with respect to $x$ . The derivative of a constant is $0$ .
Solve for $\frac{dy}{dx}$ : Combine the derivatives from the previous steps to form the differentiated equation: $18x^2 - \frac{8}{y^2} \cdot \frac{dy}{dx} = 0$ .
Simplify $\frac{dy}{dx}$ : Solve for $\frac{dy}{dx}$ . To do this, we isolate $\frac{dy}{dx}$ on one side of the equation. We get $\frac{dy}{dx} = \frac{18x^2 \cdot y^2}{8}$ .
Simplify $\frac{dy}{dx}$ : Solve for $\frac{dy}{dx}$ . To do this, we isolate $\frac{dy}{dx}$ on one side of the equation. We get $\frac{dy}{dx} = \frac{18x^2 \cdot y^2}{8}$ .Simplify the expression for $\frac{dy}{dx}$ . We can simplify $\frac{18x^2 \cdot y^2}{8}$ to $\frac{9x^2 \cdot y^2}{4}$ .

More problems from Complex conjugate theorem

Question

Find the degree of this polynomial.

\newline

`5b^{10} + 3b^3`

\newline

Posted 2 months ago

Question

\newline

(9a + 5) - (2a + 4)

\newline

Posted 2 months ago

Question

Find the product. Simplify your answer.

\newline

-3v^2(v^2 - 9)

\newline

Posted 1 month ago

Question

Find the product. Simplify your answer.

\newline

(v - 3)(4v + 1)

\newline

Posted 2 months ago

Question

Find the square. Simplify your answer.

\newline

(3y + 2)^2

\newline

Posted 2 months ago

Question

Divide. If there is a remainder, include it as a simplified fraction.

\newline

(24t^2 + 36t) \div 6t

\newline

Posted 2 months ago

Question

Is the function

q(x) = x^6 - 9

even, odd, or neither?

\newline

\newline

\text{[[even][odd][neither]]}

Posted 2 months ago

Question

Find the product. Simplify your answer.

\newline

-3q^2(-3q^2 + q)

\newline

Posted 2 months ago

Question

Find the product. Simplify your answer.

\newline

(r + 3)(4r + 2)

\newline

Posted 2 months ago

Question

Find the roots of the factored polynomial.

\newline

(x + 7)(x + 4)

\newline

Write your answer as a list of values separated by commas.

\newline

x =

Posted 2 months ago