Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

In /_\IJK,m/_I=(x+6)^(@),m/_J=(2x+16)^(@), and m/_K=(6x+14)^(@). What is the value of x ? Answer:

In △IJK,m∠I=(x+6)∘,m∠J=(2x+16)∘ \triangle \mathrm{IJK}, \mathrm{m} \angle I=(x+6)^{\circ}, \mathrm{m} \angle J=(2 x+16)^{\circ} , and m∠K=(6x+14)∘ \mathrm{m} \angle K=(6 x+14)^{\circ} . What is the value of x x ?\newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Grade 8right chevron icon
Solve equations with variables on both sides: fractional coefficientsright chevron icon

Full solution

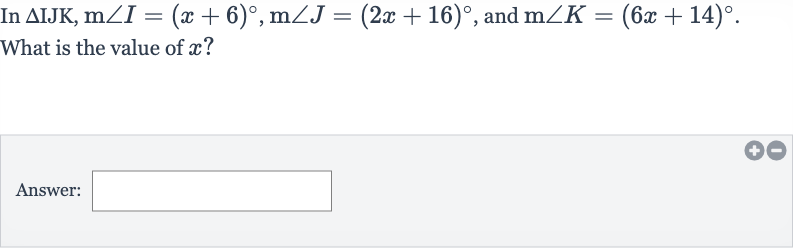
Q. In △IJK,m∠I=(x+6)∘,m∠J=(2x+16)∘ \triangle \mathrm{IJK}, \mathrm{m} \angle I=(x+6)^{\circ}, \mathrm{m} \angle J=(2 x+16)^{\circ} , and m∠K=(6x+14)∘ \mathrm{m} \angle K=(6 x+14)^{\circ} . What is the value of x x ?\newlineAnswer:
  1. Set up equation: In a triangle, the sum of the angles is always 180180 degrees. We can set up an equation with the given angle measures to find xx.
    m/I+m/J+m/K=180∘m/_{I} + m/_{J} + m/_{K} = 180^{\circ}
    (x+6)∘+(2x+16)∘+(6x+14)∘=180∘(x+6)^{\circ} + (2x+16)^{\circ} + (6x+14)^{\circ} = 180^{\circ}
  2. Combine like terms: Combine like terms to simplify the equation.\newlinex+6+2x+16+6x+14=180x + 6 + 2x + 16 + 6x + 14 = 180\newline9x+36=1809x + 36 = 180
  3. Isolate terms with x: Subtract 3636 from both sides to isolate the terms with xx.\newline9x+36−36=180−369x + 36 - 36 = 180 - 36\newline9x=1449x = 144
  4. Solve for x: Divide both sides by 99 to solve for x.\newline9x9=1449\frac{9x}{9} = \frac{144}{9}\newlinex=16x = 16

More problems from Solve equations with variables on both sides: fractional coefficients

Question
Write the sentence as an equation. \newline138 138 is equal to the quotient of w w and 30 30 \newlineType a slash ( / / ) if you want to use a division sign. \newline_____
Get tutor helpright-arrow

Posted 3 months ago

Question
Solve for x. \newline(27)x=−14(\frac{2}{7})x= -14 \newline x=x = ______
Get tutor helpright-arrow

Posted 4 months ago

Question
(85)x=32(\frac{8}{5})x= 32
Get tutor helpright-arrow

Posted 4 months ago

Question
Simplify. \newline2+22+2 \newline ______
Get tutor helpright-arrow

Posted 4 days ago

Question
Graph −27x+18y=54-27x+18y=54.
Get tutor helpright-arrow

Posted 4 months ago

Question
Evaluate.\newline48.5−0.434=48.5-0.434=
Get tutor helpright-arrow

Posted 4 months ago

Question
Evaluate.\newline96.7−0.163=96.7-0.163=
Get tutor helpright-arrow

Posted 4 months ago

Question
Evaluate.\newline4.43−0.283=4.43-0.283=
Get tutor helpright-arrow

Posted 4 months ago

Question
Evaluate.\newline3.05−0.338=3.05-0.338=
Get tutor helpright-arrow

Posted 4 months ago

Question
Evaluate.\newline6.168−0.432=6.168-0.432=
Get tutor helpright-arrow

Posted 4 months ago

View all (11)