AI tutor
Full solution
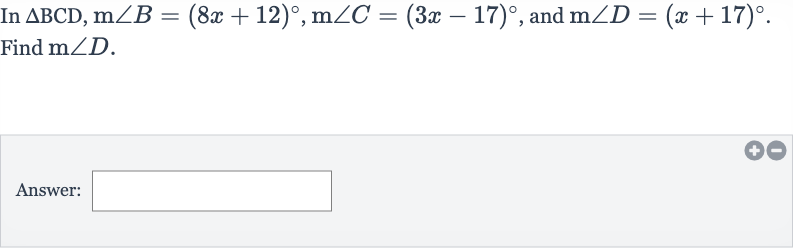
Q. In , and . Find .Answer:
- Recognize Triangle Angle Sum: Recognize that the sum of the angles in any triangle is degrees.
- Write Equation for Triangle BCD: Write an equation that represents the sum of the angles in triangle BCD. degrees
- Combine Like Terms: Combine like terms in the equation.
- Isolate Terms with x: Subtract from both sides of the equation to isolate the terms with .
- Solve for x: Divide both sides of the equation by to solve for x.
- Find Measure of Angle D: Substitute the value of back into the expression for to find its measure. degrees
More problems from Solve multi-step equations with fractional coefficients
QuestionGet tutor help