Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

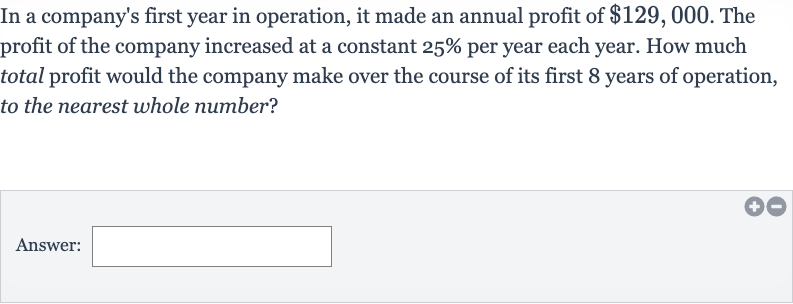
In a company's first year in operation, it made an annual profit of $129,000. The profit of the company increased at a constant 25% per year each year. How much total profit would the company make over the course of its first 8 years of operation, to the nearest whole number? Answer:

In a company's first year in operation, it made an annual profit of $129,000 \$ 129,000 . The profit of the company increased at a constant 25% 25 \% per year each year. How much total profit would the company make over the course of its first 88 years of operation, to the nearest whole number?\newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Exponential growth and decay: word problemsright chevron icon

Full solution

Q. In a company's first year in operation, it made an annual profit of $129,000 \$ 129,000 . The profit of the company increased at a constant 25% 25 \% per year each year. How much total profit would the company make over the course of its first 88 years of operation, to the nearest whole number?\newlineAnswer:
  1. Determine initial profit and rate: Determine the initial profit and the annual increase rate.\newlineThe initial profit is $129,000\$129,000, and the profit increases by 25%25\% each year.
  2. Calculate profit for each year: Calculate the profit for each year using the formula for compound interest, which is P=P0×(1+r)nP = P_0 \times (1 + r)^n, where P0P_0 is the initial amount, rr is the rate of increase, and nn is the number of years.\newlineFor the first year, the profit is $129,000\$129,000.
  3. Calculate profit for second year: Calculate the profit for the second year.\newlineThe profit for the second year would be $129,000×(1+0.25)=$129,000×1.25\$129,000 \times (1 + 0.25) = \$129,000 \times 1.25.
  4. Perform calculation for second year: Perform the calculation for the second year.$129,000×1.25=$161,250.\$129,000 \times 1.25 = \$161,250.
  5. Calculate profit for subsequent years: Continue this process to calculate the profit for each subsequent year up to the eighth year. We will use the formula P=P0×(1+r)nP = P_0 \times (1 + r)^n for each year, incrementing nn by 11 each time.
  6. Calculate total profit over 88 years: Calculate the total profit over the 88 years by adding the profit of each year.\newlineThis requires calculating the profit for each year and then summing them up.
  7. Use loop or summation formula: Use a loop or a summation formula to calculate the total profit. Since this is a geometric series, we can use the formula for the sum of a geometric series, which is S=P0×[1−(1+r)n1−(1+r)]S = P_0 \times \left[\frac{1 - (1 + r)^n}{1 - (1 + r)}\right], where SS is the sum, P0P_0 is the initial amount, rr is the rate of increase, and nn is the number of terms.
  8. Substitute values into formula: Substitute the values into the formula to find the total profit. S=$129,000×[1−(1+0.25)81−(1+0.25)]S = \$129,000 \times \left[\frac{1 - (1 + 0.25)^8}{1 - (1 + 0.25)}\right].
  9. Perform calculation: Perform the calculation.\newlineS=$129,000×[(1−1.258)(1−1.25)]S = \$129,000 \times \left[\frac{(1 - 1.25^8)}{(1 - 1.25)}\right].
  10. Calculate sum using calculator: Calculate the sum using a calculator or software to ensure accuracy.\newlineS=$129,000×[(1−1.258)(−0.25)]S = \$129,000 \times \left[\frac{(1 - 1.25^8)}{(-0.25)}\right].
  11. Calculate value of 1.2581.25^8: Calculate the value of 1.2581.25^8.\newline1.258=5.9604644775390631.25^8 = 5.960464477539063.
  12. Substitute value into sum formula: Substitute this value into the sum formula.\newlineS=$129,000×[(1−5.960464477539063)(−0.25)]S = \$129,000 \times \left[\frac{(1 - 5.960464477539063)}{(-0.25)}\right].
  13. Calculate numerator of fraction: Calculate the numerator of the fraction. 1−5.960464477539063=−4.9604644775390631 - 5.960464477539063 = -4.960464477539063.
  14. Calculate total sum: Calculate the total sum.\newlineS=$129,000×[−4.960464477539063−0.25]S = \$129,000 \times \left[\frac{-4.960464477539063}{-0.25}\right].
  15. Perform final calculation: Perform the final calculation.\newlineS=$129,000×19.84185791015625.S = \$129,000 \times 19.84185791015625.
  16. Calculate total profit: Calculate the total profit to the nearest whole number.\newlineS≈$(129,000×19.84185791015625)≈$2,559,599.57S \approx \$(129,000 \times 19.84185791015625) \approx \$2,559,599.57.

More problems from Exponential growth and decay: word problems

Question
Use the following function rule to find f(2) f(2) .\newlinef(x)=11⋅(9)x+8 f(x) = 11 \cdot (9)^x + 8 \newlinef(2)= f(2) = _____
Get tutor helpright-arrow

Posted 5 months ago

Question
Raymond works for a pharmaceutical company that is testing the effectiveness of a new medication. He gave one of the study participants 400400 milligrams of the medication, and he knows the amount remaining in the participant's body will decrease by a factor of 1/31/3 each hour.\newlineWrite an exponential equation in the form y=a(b)xy = a(b)^x that can model the amount of medication, yy, remaining in the participant's body after xx hours.\newlineUse whole numbers, decimals, or simplified fractions for the values of aa and bb.\newliney=y = ______\newline
Get tutor helpright-arrow

Posted 8 months ago

Question
A colony of 100100 bacteria doubles in size every 6060 hours. What will the population be 180180 hours from now??
Get tutor helpright-arrow

Posted 4 months ago

Question
Charlotte opened a savings account and deposited $800.00\$800.00 as principal. The account earns 8%8\% interest, compounded annually. What is the balance after 1010 years?\newlineUse the formula A=P(1+rn)ntA = P(1 + \frac{r}{n})^{nt}, where AA is the balance (final amount), PP is the principal (starting amount), rr is the interest rate expressed as a decimal, nn is the number of times per year that the interest is compounded, and tt is the time in years.\newlineRound your answer to the nearest cent.
Get tutor helpright-arrow

Posted 4 months ago

Question
Bob rented a car for a flat fee of `$150` and an additional `$10` per day. Write a linear function that represents the total cost, f(x)f(x), after xx days.
Get tutor helpright-arrow

Posted 10 months ago

Question
Sally purchased a laptop for `$800` and has to pay a service fee of `$20` for each day until it is delivered. Write a linear function to represent the total cost, h(x)h(x), after xx days.
Get tutor helpright-arrow

Posted 10 months ago

Question
Kevin invested $1,000\$1,000 in a savings account with a daily interest rate of 0.1%0.1\%. Write an exponential function to represent the total amount, s(x)s(x), in his account after xx days.
Get tutor helpright-arrow

Posted 9 months ago

Question
A=2450+740s A=2450+740 s \newlineThe total amount, A A , in thousands of dollars, of scholarship money given out by a college throughout its history at a time of s s semesters after the summer of the year 20002000 is given by the equation. What was the total amount of scholarship money, in thousands of dollars, given out by the college up to the summer of the year 20002000 ?
Get tutor helpright-arrow

Posted 5 months ago

Question
T=235+980d T=235+980 d \newlineThe torque, T T , in newton-meters, on a diving board with a 100100 kilogram weight placed d d meters from the diving board's fulcrum is given by the equation. How much torque in newton-meters is on the diving board when the weight is placed at the fulcrum?
Get tutor helpright-arrow

Posted 8 months ago

Question
R=42−0.7t R=42-0.7 t \newlineQuinn returned home one summer's day to find it extremely hot. He turned the air conditioner on, and the room's temperature began decreasing at a constant rate. The given equation shown gives the room's temperature, R R , in degrees Celsius, t t minutes after Quinn turned on the air conditioner. What was the room's temperature, in degrees Celsius, when Quinn returned home?
Get tutor helpright-arrow

Posted 7 months ago

View all (161)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant