Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

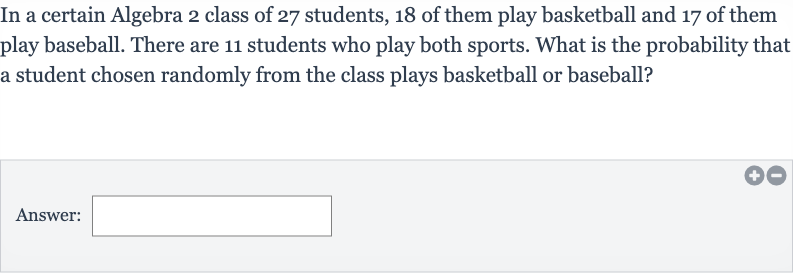
In a certain Algebra 2 class of 27 students, 18 of them play basketball and 17 of them play baseball. There are 11 students who play both sports. What is the probability that a student chosen randomly from the class plays basketball or baseball?
Answer:

In a certain Algebra $2$ class of $27$ students, $18$ of them play basketball and $17$ of them play baseball. There are $11$ students who play both sports. What is the probability that a student chosen randomly from the class plays basketball or baseball? $\newline$ Answer:

View step-by-step help

View step-by-step help

Probability of simple events

Full solution

Q. In a certain Algebra

2

class of

27

students,

18

of them play basketball and

17

of them play baseball. There are

11

students who play both sports. What is the probability that a student chosen randomly from the class plays basketball or baseball?

\newline

Answer:

Use Inclusion-Exclusion Principle: To find the probability that a student plays basketball or baseball, we need to use the principle of inclusion-exclusion. The formula for the probability of $A$ or $B$ is $P(A) + P(B) - P(A \text{ and } B)$ .
Calculate $P(\text{Basketball})$ : First, we calculate the probability of a student playing basketball. $P(\text{Basketball}) = \frac{\text{Number of students playing basketball}}{\text{Total number of students}}.$ $P(\text{Basketball}) = \frac{18}{27}.$
Calculate $P(\text{Baseball})$ : Next, we calculate the probability of a student playing baseball. $P(\text{Baseball}) = \frac{\text{Number of students playing baseball}}{\text{Total number of students}}.$ $\newline$ $P(\text{Baseball}) = \frac{17}{27}.$
Calculate $P(\text{Basketball and Baseball})$ : Then, we calculate the probability of a student playing both basketball and baseball. $P(\text{Basketball and Baseball}) = \frac{\text{Number of students playing both sports}}{\text{Total number of students}}.$ $P(\text{Basketball and Baseball}) = \frac{11}{27}.$
Apply Inclusion-Exclusion Principle: Now, we apply the principle of inclusion-exclusion to find the probability of a student playing basketball or baseball. $\newline$ $P(\text{Basketball or Baseball}) = P(\text{Basketball}) + P(\text{Baseball}) - P(\text{Basketball and Baseball})$ . $\newline$ $P(\text{Basketball or Baseball}) = (\frac{18}{27}) + (\frac{17}{27}) - (\frac{11}{27})$ .
Simplify the Expression: We simplify the expression to find the final probability. $\newline$ $P(\text{Basketball or Baseball}) = (18 + 17 - 11) / 27$ . $\newline$ $P(\text{Basketball or Baseball}) = 24 / 27$ .
Final Probability: Finally, we can simplify the fraction $\frac{24}{27}$ by dividing both the numerator and the denominator by their greatest common divisor, which is $3$ .
$P(\text{Basketball or Baseball}) = \frac{(24 \div 3)}{(27 \div 3)}.$
$P(\text{Basketball or Baseball}) = \frac{8}{9}.$

More problems from Probability of simple events

Question

6

-sided die two times. What is the probability of rolling a number greater than \(3\) and then rolling a number less than \(4\)? Write your answer as a percentage. ____ `%`

Posted 1 month ago

Question

9

college students running for student government class president. The candidates include

7

education majors.

\newline

6

of the candidates are randomly chosen to give the first

6

speeches, what is the probability that all of them are education majors?

\newline

Write your answer as a decimal rounded to four decimal places.

Posted 2 months ago

Question

In an experiment, the probability that event

A

\frac{2}{7}

, the probability that event

B

\frac{7}{9}

, and the probability that events

A

B

\frac{1}{9}

\newline

A

B

independent events?

\newline

\newline

\text{yes}

\newline

\text{no}

Posted 2 months ago

Question

At a science museum, visitors can compete to see who has a faster reaction time. Competitors watch a red screen, and the moment they see it turn from red to green, they push a button. The machine records their reaction times and also asks competitors to report their gender.

\newline

The probability that a competitor reacted in over

0.7

0.6

, the probability that a competitor was female is

0.4

, and the probability that a competitor reacted in over

0.7

seconds and was female is

0.3

\newline

What is the probability that a randomly chosen competitor reacted in over

0.7

seconds or was female?

\newline

Write your answer as a whole number, decimal, or simplified fraction.

\newline

Posted 2 months ago

Question

A restaurant server claims that \(28\)% of the time he leaves candy with the bill, the customer tips well.

\newline

If the server's claim is true, and one day he leaves candy with \(4\) customers' bills, what is the probability that exactly \(3\) of those customers will tip well?

\newline

Write your answer as a decimal rounded to the nearest thousandth.

Posted 1 month ago

Question

There is a spinner with `50` equally likely sections, numbered from `1` to `50`. You have the opportunity to spin it. If the number is odd, you win $`11`. If the number is even, you win nothing. If you play the game, what is the expected payoff?\(\$\)_____

Posted 2 months ago

Question

There are two different raffles you can enter.

\newline

In raffle A, one ticket will win a `$720` prize, and the other tickets will win nothing. There are

1,000

in the raffle, each costing `$11`.

\newline

Raffle B is for a `$370` prize. Out of

125

tickets, each costing `$5`, one ticket will win the prize, and the other tickets will win nothing.

\newline

Which raffle is a better deal?

\newline

\newline

\text{[A]Raffle A}

\newline

\text{[B]Raffle B}

Posted 2 months ago

Question

X

is a normally distributed random variable with mean

49

and standard deviation

25

\newline

What is the probability that

X

24

74

?

\newline

0.68-0.95-0.997

rule and write your answer as a decimal. Round to the nearest thousandth if necessary.

\newline

Posted 2 months ago

Question

X

is a normally distributed random variable with mean

65

and standard deviation

8

\newline

What is the probability that

X

62

68

?

\newline

Write your answer as a decimal rounded to the nearest thousandth.

\newline

Posted 2 months ago

Question

Complete the statement. Round your answer to the nearest thousandth.

\newline

In a population that is normally distributed with mean

43

and standard deviation

3

30\%

of the values are those less than ______.

Posted 2 months ago