AI tutor
Full solution
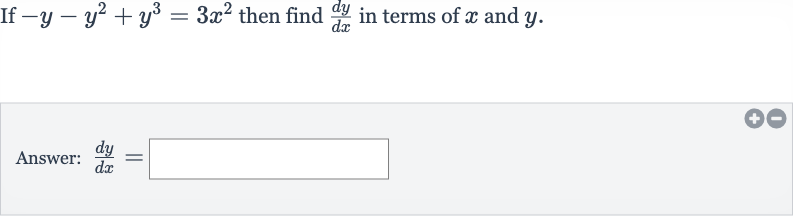
Q. If then find in terms of and .Answer:
- Identify Given Equation: Identify the given equation and the requirement to differentiate with respect to .Given equation: We need to find .
- Differentiate with Respect: Differentiate both sides of the equation with respect to , using implicit differentiation.
- Apply Chain Rule: Apply the chain rule to differentiate the terms involving with respect to .
So, - Combine Like Terms: Combine like terms and solve for .
- Isolate : Isolate on one side of the equation.