AI tutor
Full solution
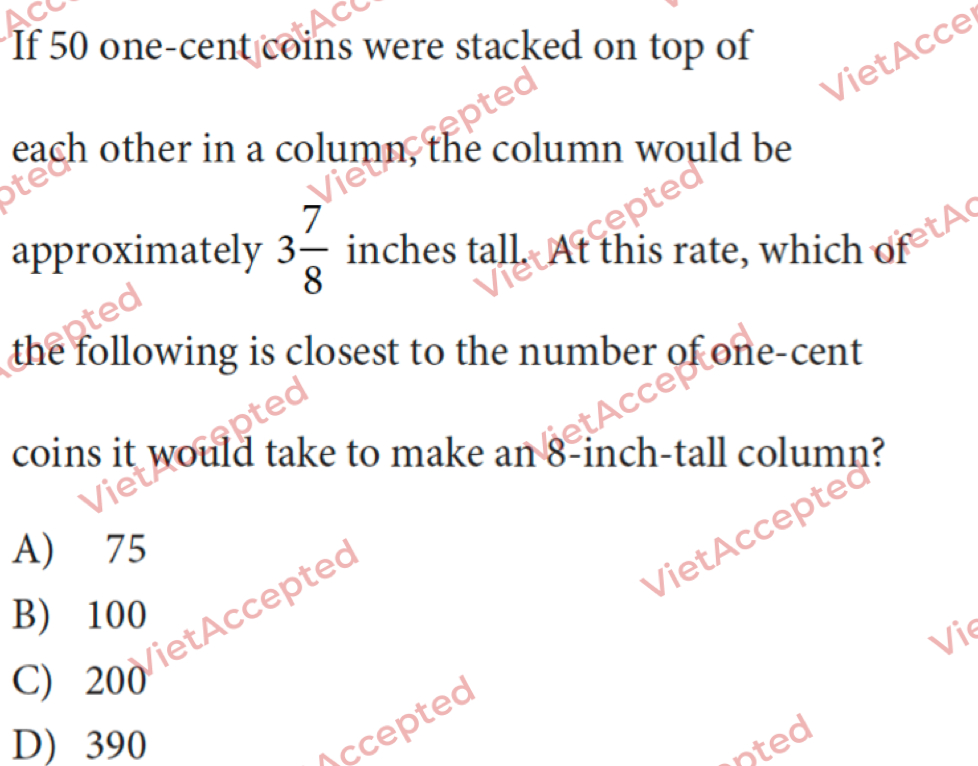
Q. If one-cent jcoins were stacked on top of each other in a column, the column would be approximately inches tall. At this rate, which of the following is closest to the number of one-cent coins it would take to make an -inch-tall column?A) B) C) D)
- Calculate coin height: Calculate the height of one coin.Height of coins = inches.Convert to an improper fraction: inches.Height of one coin = inches per coin.
- Calculate coins for -inch column: Calculate the number of coins needed for an -inch column.Number of coins = Simplify the division: .
- Round to nearest option: Round to the nearest option.The closest options are or .Since is closer to , choose .