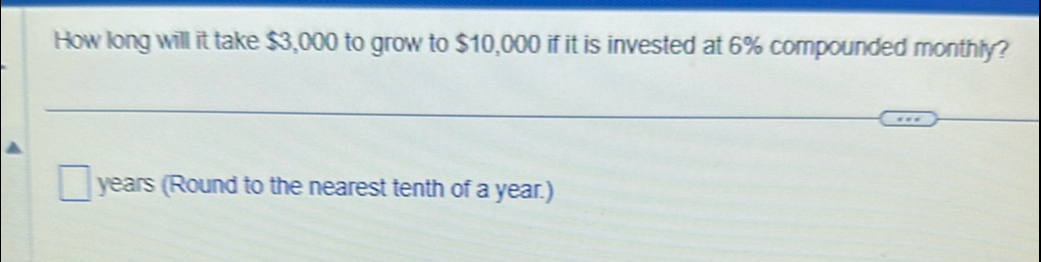
How long will it take $3,000 to grow to $10,000 if it is invested at 6% compounded monthly? ◻ years (Round to the nearest tenth of a year.)

Question

How long will it take  $3,000 to grow to  $10,000 if it is invested at  6% compounded monthly?  ◻ years (Round to the nearest tenth of a year.)

Bytelearn · Accepted Answer

Identify Variables: Identify the variables from the problem. Principal amount (P) = $3,000 Future value (A) = $10,000 Annual interest rate (r) = 6% or 0.06 (as a decimal) Compounded monthly means the number of compounding periods per year (n) = 12 We need to find the time (t) in years.Use Formula: Use the compound interest formula to set up the equation. The compound interest formula is A = P(1 + r/n)^(nt). Substitute the given values into the formula to get: $10,000 = $3,000(1 + 0.06/12)^(12t).Simplify Equation: Simplify the equation. $10,000 = $3,000(1 + 0.005)^(12t) $10,000 = $3,000(1.005)^(12t)Isolate Exponential Part: Divide both sides of the equation by $3,000 to isolate the exponential part. $10,000 / $3,000 = (1.005)^(12t) 3.3333... = (1.005)^(12t)Take Natural Logarithm: Take the natural logarithm (ln) of both sides to solve for the exponent. ln(3.3333...) = ln((1.005)^(12t)) ln(3.3333...) = 12t * ln(1.005)Solve for Exponent: Divide both sides by 12 * ln(1.005) to solve for t. t = ln(3.3333...) / (12 * ln(1.005))Calculate Value of t: Calculate the value of t using a calculator. t ≈ ln(3.3333...) / (12 * ln(1.005)) t ≈ 1.0986122886681098 / (12 * 0.0049875621120890275) t ≈ 1.0986122886681098 / 0.05985074534506833 t ≈ 18.354648862953Convert to Years: Convert the time from months to years by dividing by 12. t ≈ 18.354648862953 / 12 t ≈ 1.52955407191275 Round to the nearest tenth of a year. t ≈ 1.5 years

How long will it take $\$ 3,000$ to grow to $\$ 10,000$ if it is invested at $6 \%$ compounded monthly? $\newline$ $\square$ years (Round to the nearest tenth of a year.)

Full solution

More problems from Finance problems

Related topics

How long will it take $3,000 \$ 3,000 $3,000 to grow to $10,000 \$ 10,000 $10,000 if it is invested at 6% 6 \% 6% compounded monthly?\newline□ \square □ years (Round to the nearest tenth of a year.)

How long will it take $\$ 3,000$ to grow to $\$ 10,000$ if it is invested at $6 \%$ compounded monthly? $\newline$ $\square$ years (Round to the nearest tenth of a year.)