AI tutor
Full solution
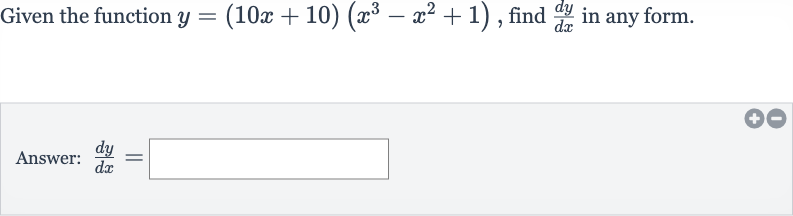
Q. Given the function , find in any form.Answer:
- Identify function: Identify the function to differentiate.We are given the function and we need to find its derivative with respect to , which is denoted as .
- Apply product rule: Apply the product rule for differentiation.The product rule states that the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function. Let's denote and . Then, .
- Differentiate : Differentiate with respect to . The derivative of with respect to is .
- Differentiate : Differentiate with respect to . The derivative of with respect to is .
- Substitute into formula: Substitute , , and into the product rule formula..
- Expand and simplify: Expand the terms and simplify the expression..
- Combine like terms: Combine like terms.