Full solution
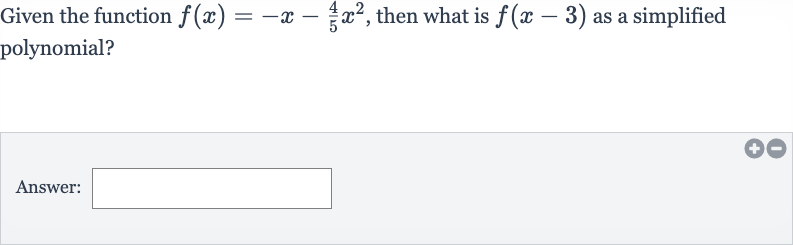
Q. Given the function , then what is as a simplified polynomial?Answer:
- Understand function transformation: Understand the function transformation.We need to find , which means we will replace every instance of in the function with .
- Apply transformation to function: Apply the transformation to the function.
- Expand terms in transformed function: Expand the terms in the transformed function.
- Distribute negative sign and fraction: Distribute the negative sign and the fraction across the terms.
- Combine like terms: Combine like terms, if any, to simplify the polynomial.There are no like terms to combine, so the polynomial is already in its simplest form.
More problems from Composition of linear and quadratic functions: find an equation
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help