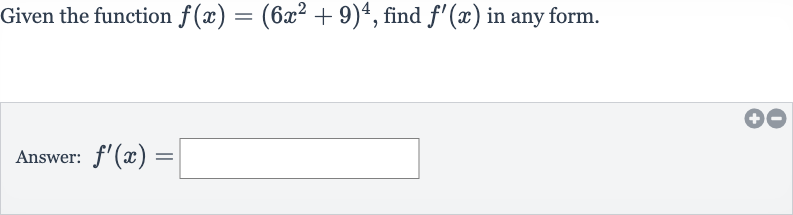
Given the function f(x)=(6x^(2)+9)^(4), find f^(')(x) in any form. Answer: f^(')(x)=

Question

Given the function  f(x)=(6x^(2)+9)^(4), find  f^(')(x) in any form. Answer:  f^(')(x)=

Bytelearn · Accepted Answer

Identify function & operation: Identify the function and the operation needed. We need to find the derivative of the function f(x)=(6x^(2)+9)^(4). This requires using the chain rule for differentiation.Apply chain rule: Apply the chain rule. The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function. In this case, the outer function is u^4 and the inner function is 6x^2 + 9.Differentiate outer function: Differentiate the outer function with respect to the inner function. Let u = 6x^2 + 9. Then the outer function is u^4. The derivative of u^4 with respect to u is 4u^3.Differentiate inner function: Differentiate the inner function with respect to x. The derivative of 6x^2 with respect to x is 12x, and the derivative of 9 with respect to x is 0. So the derivative of the inner function 6x^2 + 9 with respect to x is 12x.Combine derivatives: Combine the derivatives using the chain rule. Now we multiply the derivative of the outer function by the derivative of the inner function to get the derivative of the composite function. f'(x) = 4u^3 * 12xSubstitute back into equation: Substitute u back into the equation. Replace u with 6x^2 + 9 in the derivative. f'(x) = 4(6x^2 + 9)^3 * 12xSimplify expression: Simplify the expression. We can simplify the expression by multiplying the constants and combining like terms. f'(x) = 48x(6x^2 + 9)^3

Given the function $f(x)=\left(6 x^{2}+9\right)^{4}$ , find $f^{\prime}(x)$ in any form. $\newline$ Answer: $f^{\prime}(x)=$

Full solution

More problems from Evaluate exponential functions

Related topics

Given the function f(x)=(6x2+9)4 f(x)=\left(6 x^{2}+9\right)^{4} f(x)=(6x2+9)4, find f′(x) f^{\prime}(x) f′(x) in any form.\newlineAnswer: f′(x)= f^{\prime}(x)= f′(x)=

Given the function $f(x)=\left(6 x^{2}+9\right)^{4}$ , find $f^{\prime}(x)$ in any form. $\newline$ Answer: $f^{\prime}(x)=$