Full solution
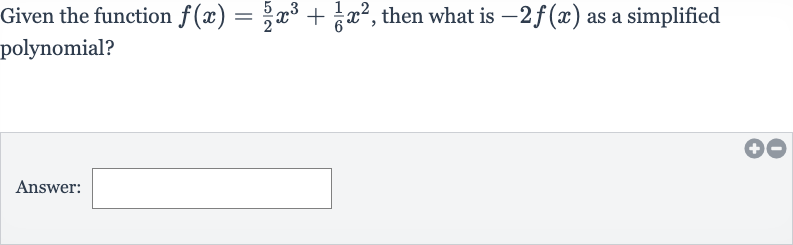
Q. Given the function , then what is as a simplified polynomial?Answer:
- Multiply by : We are given the function . To find , we need to multiply the entire function by .
- Distribute : Now, distribute the to both terms in the function.
- Simplify terms: Simplify each term by multiplying the coefficients.
- Final result: The polynomial is now simplified, and there is no need for further simplification.So,
More problems from Composition of linear and quadratic functions: find an equation
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help