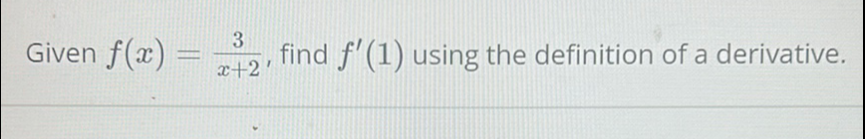
Given f(x)=(3)/(x+2), find f^(')(1) using the definition of a derivative.

Question

Given  f(x)=(3)/(x+2), find  f^(')(1) using the definition of a derivative.

Bytelearn · Accepted Answer

Given Function: We are given the function f(x) = 3/(x+2) and we need to find its derivative at x = 1 using the definition of a derivative. The definition of a derivative is: f'(x) = lim(h -> 0) [f(x+h) - f(x)] / h Let's apply this definition to our function.Substitute x+h: First, we need to find the expression for f(x+h). This means we will substitute (x+h) for x in the function f(x): f(x+h) = 3/((x+h)+2) = 3/(x+h+2)Plug into Derivative Definition: Now, we will plug f(x+h) and f(x) into the definition of the derivative: f'(x) = lim(h -> 0) [3/(x+h+2) - 3/(x+2)] / hFind Common Denominator: Next, we need to find a common denominator for the terms in the numerator to combine them: The common denominator is (x+h+2)(x+2), so we rewrite the numerator: f'(x) = lim(h -> 0) [(3(x+2) - 3(x+h+2)) / ((x+h+2)(x+2))] / hSimplify Numerator: Now, we simplify the numerator: 3(x+2) - 3(x+h+2) = 3x + 6 - 3x - 3h - 6 = -3h So the expression becomes: f'(x) = lim(h -> 0) [-3h / ((x+h+2)(x+2) * h)]Cancel out h: We can now cancel out the h in the numerator and denominator: f'(x) = lim(h -> 0) [-3 / ((x+h+2)(x+2))]Take the Limit: Now we can take the limit as h approaches 0: f'(x) = -3 / ((x+2)(x+2))Substitute x=1: Finally, we substitute x = 1 into the derivative to find f'(1): f'(1) = -3 / ((1+2)(1+2)) = -3 / (3*3) = -3 / 9 = -1/3

Given $f(x)=\frac{3}{x+2}$ , find $f^{\prime}(1)$ using the definition of a derivative.

Full solution

More problems from Evaluate expression when two complex numbers are given

Related topics

Given f(x)=3x+2 f(x)=\frac{3}{x+2} f(x)=x+23​, find f′(1) f^{\prime}(1) f′(1) using the definition of a derivative.

Given $f(x)=\frac{3}{x+2}$ , find $f^{\prime}(1)$ using the definition of a derivative.