AI tutor
Full solution
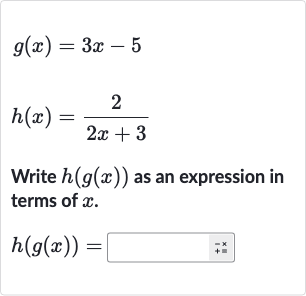
Q. Write as an expression in terms of .
- Substitute into : First, we need to substitute the expression for into to find . The function is given as , so we will replace every in with .
- Simplify expression: The function is . Substituting into , we get .
- Simplify expression inside parentheses: Now, we simplify the expression inside the parentheses: .
- Further simplification: Simplifying further, we get .
- Write complete expression for : Now, we can write the complete expression for : .
More problems from Find the inverse of a linear function
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help