Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

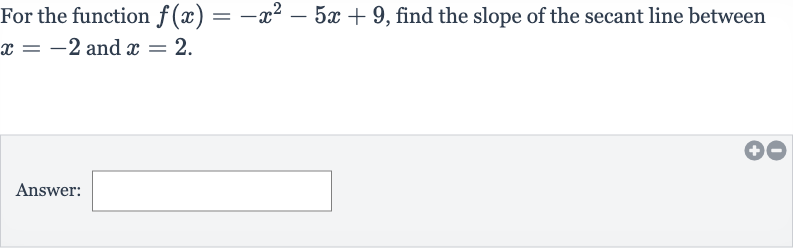
For the function
f(x)=-x^(2)-5x+9, find the slope of the secant line between
x=-2 and
x=2.
Answer:

For the function $f(x)=-x^{2}-5 x+9$ , find the slope of the secant line between $x=-2$ and $x=2$ . $\newline$ Answer:

View step-by-step help

View step-by-step help

Find the roots of factored polynomials

Full solution

Q. For the function

f(x)=-x^{2}-5 x+9

, find the slope of the secant line between

x=-2

and

x=2

.

\newline

Answer:

Calculate $f(-2)$ : To find the slope of the secant line between two points on a function, we use the formula for slope, which is the change in $y$ divided by the change in $x$ , or $\frac{f(x_2) - f(x_1)}{x_2 - x_1}$ . We need to calculate the function values at $x = -2$ and $x = 2$ .
Calculate $f(2)$ : First, we calculate $f(-2)$ . Plugging $-2$ into the function, we get $f(-2) = -(-2)^2 - 5(-2) + 9 = -4 + 10 + 9$ .
Find slope formula: Simplifying $f(-2)$ , we get $f(-2) = -4 + 10 + 9 = 15$ .
Calculate slope: Next, we calculate $f(2)$ . Plugging $2$ into the function, we get $f(2) = -(2)^2 - 5(2) + 9 = -4 - 10 + 9$ .
Calculate slope: Next, we calculate $f(2)$ . Plugging $2$ into the function, we get $f(2) = -(2)^2 - 5(2) + 9 = -4 - 10 + 9$ . Simplifying $f(2)$ , we get $f(2) = -4 - 10 + 9 = -5$ .
Calculate slope: Next, we calculate $f(2)$ . Plugging $2$ into the function, we get $f(2) = -(2)^2 - 5(2) + 9 = -4 - 10 + 9$ . Simplifying $f(2)$ , we get $f(2) = -4 - 10 + 9 = -5$ . Now we have the two points $(-2, f(-2))$ and $(2, f(2))$ , which are $(-2, 15)$ and $(2, -5)$ . We can use these to find the slope of the secant line: $\text{slope} = \frac{f(2) - f(-2)}{2 - (-2)}$ .
Calculate slope: Next, we calculate $f(2)$ . Plugging $2$ into the function, we get $f(2) = -(2)^2 - 5(2) + 9 = -4 - 10 + 9$ . Simplifying $f(2)$ , we get $f(2) = -4 - 10 + 9 = -5$ . Now we have the two points $(-2, f(-2))$ and $(2, f(2))$ , which are $(-2, 15)$ and $(2, -5)$ . We can use these to find the slope of the secant line: $\text{slope} = \frac{f(2) - f(-2)}{2 - (-2)}$ . Plugging in the values, we get $2$ $0$ .
Calculate slope: Next, we calculate $f(2)$ . Plugging $2$ into the function, we get $f(2) = -(2)^2 - 5(2) + 9 = -4 - 10 + 9$ . Simplifying $f(2)$ , we get $f(2) = -4 - 10 + 9 = -5$ . Now we have the two points $(-2, f(-2))$ and $(2, f(2))$ , which are $(-2, 15)$ and $(2, -5)$ . We can use these to find the slope of the secant line: $\text{slope} = \frac{f(2) - f(-2)}{2 - (-2)}$ . Plugging in the values, we get $2$ $0$ . Simplifying the slope, we get $2$ $1$ .

More problems from Find the roots of factored polynomials

Question

Find the degree of this polynomial.

\newline

`5b^{10} + 3b^3`

\newline

Posted 5 months ago

Question

\newline

(9a + 5) - (2a + 4)

\newline

Posted 5 months ago

Question

Find the product. Simplify your answer.

\newline

-3v^2(v^2 - 9)

\newline

Posted 5 months ago

Question

Find the product. Simplify your answer.

\newline

(v - 3)(4v + 1)

\newline

Posted 5 months ago

Question

Find the square. Simplify your answer.

\newline

(3y + 2)^2

\newline

Posted 5 months ago

Question

Divide. If there is a remainder, include it as a simplified fraction.

\newline

(24t^2 + 36t) \div 6t

\newline

Posted 5 months ago

Question

Is the function

q(x) = x^6 - 9

even, odd, or neither?

\newline

\newline

\text{[[even][odd][neither]]}

Posted 9 months ago

Question

Find the product. Simplify your answer.

\newline

-3q^2(-3q^2 + q)

\newline

Posted 9 months ago

Question

Find the product. Simplify your answer.

\newline

(r + 3)(4r + 2)

\newline

Posted 9 months ago

Question

Find the roots of the factored polynomial.

\newline

(x + 7)(x + 4)

\newline

Write your answer as a list of values separated by commas.

\newline

x =

Posted 9 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant