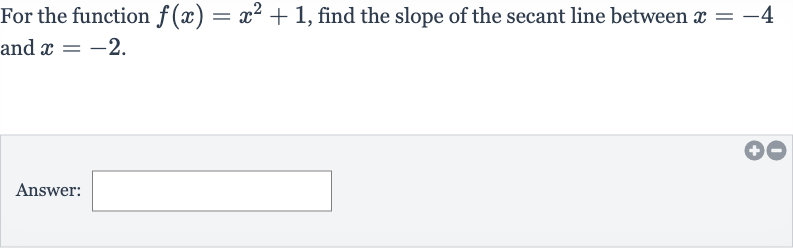
For the function f(x)=x^(2)+1, find the slope of the secant line between x=-4 and x=-2. Answer:

Question

For the function  f(x)=x^(2)+1, find the slope of the secant line between  x=-4 and  x=-2. Answer:

Bytelearn · Accepted Answer

Identify Points: To find the slope of the secant line between two points on a function, we use the slope formula, which is the change in y divided by the change in x (rise over run). The two points we are interested in are when x = -4 and x = -2. We need to find the corresponding y values for these x values by plugging them into the function f(x) = x^2 + 1.Calculate Y Values: First, let's find the y value when x = -4. We substitute -4 into the function: f(-4) = (-4)^2 + 1 = 16 + 1 = 17.Calculate Slope: Next, we find the y value when x = -2. We substitute -2 into the function: f(-2) = (-2)^2 + 1 = 4 + 1 = 5.Substitute into Formula: Now we have two points on the function: Point A (-4, 17) and Point B (-2, 5). We can use these points to calculate the slope of the secant line. The slope (m) is given by the formula m = (y2 - y1) / (x2 - x1), where (x1, y1) and (x2, y2) are the coordinates of the two points.Substitute the coordinates of the two points into the slope formula: m = (5 - 17) / (-2 - (-4)) = (-12) / (2) = -6.

For the function $f(x)=x^{2}+1$ , find the slope of the secant line between $x=-4$ and $x=-2$ . $\newline$ Answer:

Full solution

More problems from Find the roots of factored polynomials

Related topics

For the function f(x)=x2+1 f(x)=x^{2}+1 f(x)=x2+1, find the slope of the secant line between x=−4 x=-4 x=−4 and x=−2 x=-2 x=−2.\newlineAnswer:

For the function $f(x)=x^{2}+1$ , find the slope of the secant line between $x=-4$ and $x=-2$ . $\newline$ Answer: