AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
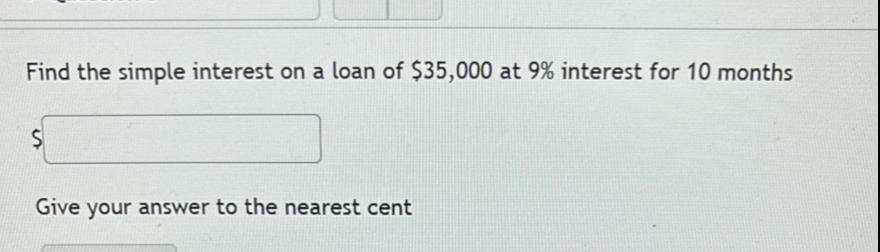
Find the simple interest on a loan of at interest for months ___________Give your answer to the nearest cent
Full solution
Q. Find the simple interest on a loan of at interest for months ___________Give your answer to the nearest cent
- Understand formula for simple interest: Understand the formula for simple interest. Simple interest can be calculated using the formula , where is the interest, is the principal amount, is the annual interest rate (in decimal form), and is the time in years.
- Convert annual interest rate to decimal: Convert the annual interest rate to a decimal.The annual interest rate is , so as a decimal, .
- Convert time from months to years: Convert the time from months to years.Since there are months in a year, months is years. So,
- Calculate simple interest using values: Calculate the simple interest using the values.Now we have , , and . Plugging these into the formula gives us .
- Perform the calculation: Perform the calculation. (approximately)
- Round result to nearest cent: Round the result to the nearest cent.The interest calculated is approximately . Since we are asked to give the answer to the nearest cent, we do not need to round further as the result is already in whole dollars.
More problems from Exponential growth and decay: word problems
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
