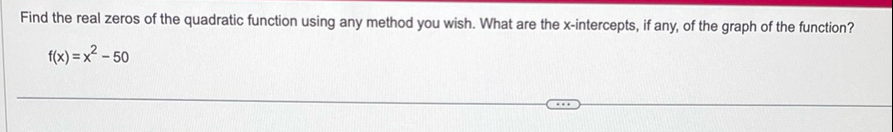
Full solution
Q. Find the real zeros of the quadratic function using any method you wish. What are the x-intercepts, if any, of the graph of the function?
- Set equation to zero: To find the real zeros of the quadratic function , we need to set the function equal to zero and solve for .
- Solve for : We can solve this equation by taking the square root of both sides. However, since we are taking the square root of a negative number, we will have two solutions, one positive and one negative.
- Square root both sides: Taking the square root of both sides gives us:
- Simplify square root: Simplifying the square root of , we get:
- Identify real zeros: Therefore, the real zeros of the function are and . These zeros are also the -intercepts of the graph of the function.