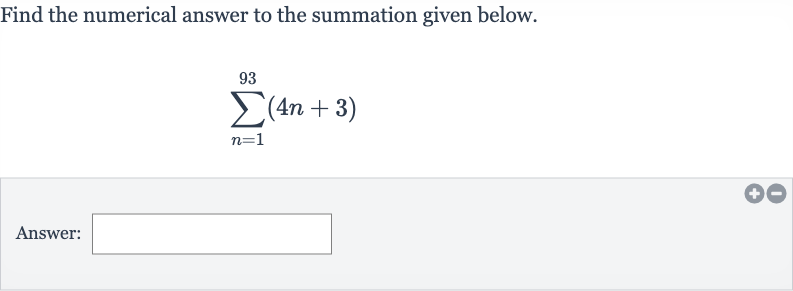
Find the numerical answer to the summation given below. sum_(n=1)^(93)(4n+3) Answer:

Question

Find the numerical answer to the summation given below.  sum_(n=1)^(93)(4n+3) Answer:

Bytelearn · Accepted Answer

Arithmetic Series Representation: The summation given is an arithmetic series where each term can be represented as 4n + 3, with n starting at 1 and going up to 93. To find the sum of this series, we can use the formula for the sum of an arithmetic series, which is S = (n/2)(a_1 + a_n), where n is the number of terms, a_1 is the first term, and a_n is the last term.Find First and Last Terms: First, we need to find the first term a_1 and the last term a_n of the series. The first term a_1 is obtained by substituting n = 1 into the expression 4n + 3, which gives us a_1 = 4(1) + 3 = 7. The last term a_n is obtained by substituting n = 93 into the expression 4n + 3, which gives us a_n = 4(93) + 3 = 372 + 3 = 375.Calculate Sum Formula: Now that we have the first and last terms, we can find the sum of the series. The number of terms n is 93 since we are summing from n = 1 to n = 93. Using the formula S = (n/2)(a_1 + a_n), we get S = (93/2)(7 + 375).Perform Calculations: We can now perform the calculations. First, we add the first and last terms: 7 + 375 = 382. Then we multiply this sum by the number of terms divided by 2: S = (93/2) * 382.Final Result: To simplify the calculation, we can multiply 382 by 93, which gives us 35526, and then divide by 2: S = 35526 / 2.Finally, we perform the division: 35526 / 2 = 17763. This is the sum of the series.

Find the numerical answer to the summation given below. $\newline$ $\sum_{n=1}^{93}(4 n+3)$ $\newline$ Answer:

Full solution

More problems from Find the roots of factored polynomials

Related topics

Find the numerical answer to the summation given below.\newline∑n=193(4n+3) \sum_{n=1}^{93}(4 n+3) n=1∑93​(4n+3)\newlineAnswer:

Find the numerical answer to the summation given below. $\newline$ $\sum_{n=1}^{93}(4 n+3)$ $\newline$ Answer: