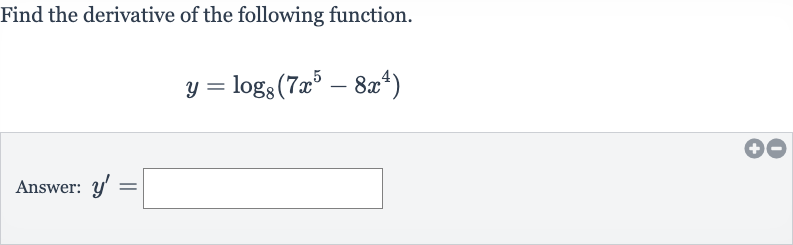
Find the derivative of the following function. y=log_(8)(7x^(5)-8x^(4)) Answer: y^(')=

Question

Find the derivative of the following function.  y=log_(8)(7x^(5)-8x^(4)) Answer:  y^(')=

Bytelearn · Accepted Answer

Apply Change of Base Formula: To find the derivative of the function y = log base 8 of (7x^5 - 8x^4), we will use the change of base formula for logarithms and the chain rule for differentiation. Change of base formula: log base a of b = log base c of b / log base c of a We will use the natural logarithm (base e) for this purpose. y = log base 8 of (7x^5 - 8x^4) can be rewritten as y = ln(7x^5 - 8x^4) / ln(8)Use Chain Rule for Differentiation: Now we need to differentiate the function with respect to x using the chain rule. The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function. Let u = 7x^5 - 8x^4, then y = ln(u) / ln(8) The derivative of ln(u) with respect to u is 1/u, and we need to multiply this by the derivative of u with respect to x.Find Derivative of Inner Function: Let's find the derivative of u = 7x^5 - 8x^4 with respect to x. Using the power rule, the derivative of x^n with respect to x is n*x^(n-1). The derivative of 7x^5 is 35x^4 and the derivative of -8x^4 is -32x^3. So, the derivative of u with respect to x is du/dx = 35x^4 - 32x^3.Apply Chain Rule to Find Derivative: Now we can apply the chain rule to find the derivative of y. y' = (1/u) * (du/dx) / ln(8) Substitute u = 7x^5 - 8x^4 and du/dx = 35x^4 - 32x^3 into the equation. y' = (1/(7x^5 - 8x^4)) * (35x^4 - 32x^3) / ln(8)Simplify the Derivative: Finally, we simplify the expression for the derivative. y' = (35x^4 - 32x^3) / ((7x^5 - 8x^4) * ln(8)) This is the derivative of the function y = log base 8 of (7x^5 - 8x^4) with respect to x.

Find the derivative of the following function. $\newline$ $y=\log _{8}\left(7 x^{5}-8 x^{4}\right)$ $\newline$ Answer: $y^{\prime}=$

Full solution

More problems from Quotient property of logarithms

Related topics

Find the derivative of the following function.\newliney=log⁡8(7x5−8x4) y=\log _{8}\left(7 x^{5}-8 x^{4}\right) y=log8​(7x5−8x4)\newlineAnswer: y′= y^{\prime}= y′=

Find the derivative of the following function. $\newline$ $y=\log _{8}\left(7 x^{5}-8 x^{4}\right)$ $\newline$ Answer: $y^{\prime}=$