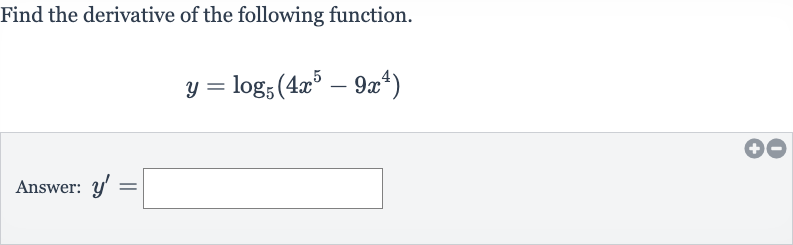
Find the derivative of the following function. y=log_(5)(4x^(5)-9x^(4)) Answer: y^(')=

Question

Find the derivative of the following function.  y=log_(5)(4x^(5)-9x^(4)) Answer:  y^(')=

Bytelearn · Accepted Answer

Identify function & derivative type: Identify the function and the type of derivative to be found. We need to find the derivative of the function y with respect to x, where y=log_5(4x^5-9x^4). This is a logarithmic differentiation problem.Apply chain rule for differentiation: Apply the chain rule for logarithmic differentiation. The chain rule states that the derivative of log_b(u(x)) is (1/u(x)) * (du/dx), where b is the base of the logarithm and u(x) is the function inside the logarithm. We also need to apply the change of base formula for logarithms because the base is 5, not e (the natural logarithm base).Change base of logarithm: Change the base of the logarithm from 5 to e. Using the change of base formula, log_5(u) = ln(u)/ln(5), where ln is the natural logarithm. So, y = ln(4x^5-9x^4)/ln(5).Differentiate using quotient rule: Differentiate the function using the quotient rule. The quotient rule states that the derivative of a function v(x)/w(x) is (v'(x)w(x) - v(x)w'(x))/(w(x))^2. However, since ln(5) is a constant, the derivative of y with respect to x is simply the derivative of ln(4x^5-9x^4) divided by ln(5).Differentiate inside function: Differentiate the inside function 4x^5-9x^4 with respect to x. The derivative of 4x^5 is 20x^4, and the derivative of -9x^4 is -36x^3. So, the derivative of 4x^5-9x^4 with respect to x is 20x^4 - 36x^3.Apply chain rule for derivative: Apply the chain rule to find the derivative of y. The derivative of y with respect to x is (1/(4x^5-9x^4)) * (20x^4 - 36x^3).Combine results from Steps 3 and 6: Combine the results from Steps 3 and 6. The derivative of y with respect to x is ((1/(4x^5-9x^4)) * (20x^4 - 36x^3))/ln(5).Simplify the expression: Simplify the expression. The derivative of y with respect to x is (20x^4 - 36x^3)/(ln(5) * (4x^5-9x^4)).

Find the derivative of the following function. $\newline$ $y=\log _{5}\left(4 x^{5}-9 x^{4}\right)$ $\newline$ Answer: $y^{\prime}=$

Full solution

More problems from Quotient property of logarithms

Related topics

Find the derivative of the following function.\newliney=log⁡5(4x5−9x4) y=\log _{5}\left(4 x^{5}-9 x^{4}\right) y=log5​(4x5−9x4)\newlineAnswer: y′= y^{\prime}= y′=

Find the derivative of the following function. $\newline$ $y=\log _{5}\left(4 x^{5}-9 x^{4}\right)$ $\newline$ Answer: $y^{\prime}=$