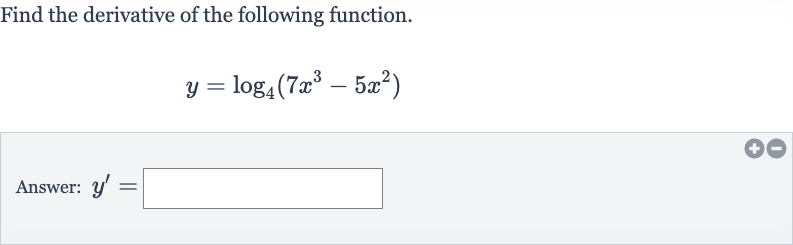
Find the derivative of the following function. y=log_(4)(7x^(3)-5x^(2)) Answer: y^(')=

Question

Find the derivative of the following function.  y=log_(4)(7x^(3)-5x^(2)) Answer:  y^(')=

Bytelearn · Accepted Answer

Apply Chain Rule and Logarithmic Differentiation: To find the derivative of the function y = log_4(7x^3 - 5x^2), we will use the chain rule and the logarithmic differentiation rule. The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function. The logarithmic differentiation rule for a logarithm with base a is given by (d/dx) log_a(u) = (1/u) * (du/dx) * (1/log(a)), where u is a function of x.Express Logarithm with Base 4 in Natural Logarithm: First, we need to express the logarithm with base 4 in terms of the natural logarithm using the change of base formula: log_a(u) = ln(u) / ln(a). So, y = log_4(7x^3 - 5x^2) can be rewritten as y = ln(7x^3 - 5x^2) / ln(4).Differentiate Quotient Using Quotient Rule: Now, we will differentiate y with respect to x using the quotient rule since y is a quotient of two functions: ln(7x^3 - 5x^2) and ln(4). The quotient rule is given by (d/dx) [v(x)/u(x)] = (u(x) * (dv/dx) - v(x) * (du/dx)) / (u(x))^2. However, since ln(4) is a constant, its derivative is 0, and the quotient rule simplifies to just the derivative of the numerator divided by the denominator.Apply Chain Rule to Differentiate Numerator: We differentiate the numerator ln(7x^3 - 5x^2) using the chain rule. The derivative of ln(u) with respect to u is 1/u, so we have (d/dx) ln(7x^3 - 5x^2) = 1/(7x^3 - 5x^2) * d/dx(7x^3 - 5x^2).Find Derivative of Inner Function: Next, we find the derivative of the inner function 7x^3 - 5x^2 with respect to x. Using the power rule, which states that the derivative of x^n with respect to x is n*x^(n-1), we get d/dx(7x^3 - 5x^2) = 21x^2 - 10x.Combine Results to Find Derivative of y: Now we can combine the results from the previous steps to find the derivative of y. We have y' = (1/(7x^3 - 5x^2)) * (21x^2 - 10x) / ln(4).Simplify Expression for y': Finally, we simplify the expression for y' by multiplying the terms. This gives us y' = (21x^2 - 10x) / ((7x^3 - 5x^2) * ln(4)).

Find the derivative of the following function. $\newline$ $y=\log _{4}\left(7 x^{3}-5 x^{2}\right)$ $\newline$ Answer: $y^{\prime}=$

Full solution

More problems from Quotient property of logarithms

Related topics

Find the derivative of the following function.\newliney=log⁡4(7x3−5x2) y=\log _{4}\left(7 x^{3}-5 x^{2}\right) y=log4​(7x3−5x2)\newlineAnswer: y′= y^{\prime}= y′=

Find the derivative of the following function. $\newline$ $y=\log _{4}\left(7 x^{3}-5 x^{2}\right)$ $\newline$ Answer: $y^{\prime}=$