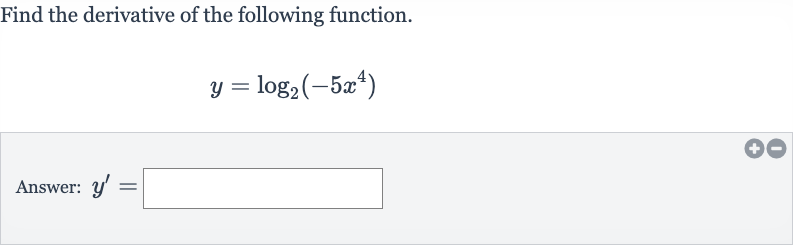
Find the derivative of the following function. y=log_(2)(-5x^(4)) Answer: y^(')=

Question

Find the derivative of the following function.  y=log_(2)(-5x^(4)) Answer:  y^(')=

Bytelearn · Accepted Answer

Identify Function and Base: Identify the function and the base of the logarithm. We have the function y = log₂(-5x⁴), where the base of the logarithm is 2. We need to find the derivative of this function with respect to x.Apply Chain Rule: Apply the chain rule for differentiation. The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function. In this case, the outer function is log₂(u) and the inner function is u = -5x⁴.Differentiate Outer Function: Differentiate the outer function. The derivative of log₂(u) with respect to u is 1/(u * ln(2)) because the change of base formula tells us that log₂(u) = ln(u)/ln(2). Therefore, the derivative of the outer function is 1/(-5x⁴ * ln(2)).Differentiate Inner Function: Differentiate the inner function. The inner function is u = -5x⁴. The derivative of -5x⁴ with respect to x is -20x³.Apply Chain Rule Multiplication: Apply the chain rule by multiplying the derivatives of the outer and inner functions. The derivative of y with respect to x is (1/(-5x⁴ * ln(2))) * (-20x³).Simplify Expression: Simplify the expression. We can simplify the expression by multiplying the constants and canceling out one x from the numerator and denominator. This gives us y' = (-20x³) / (-5x⁴ * ln(2)) = 4 / (x * ln(2)).Check Final Answer: Check for any possible math errors and write the final answer. We have followed the chain rule correctly and simplified the expression without making any algebraic errors. The final answer is y' = 4 / (x * ln(2)).

Find the derivative of the following function. $\newline$ $y=\log _{2}\left(-5 x^{4}\right)$ $\newline$ Answer: $y^{\prime}=$

Full solution

More problems from Quotient property of logarithms

Related topics

Find the derivative of the following function.\newliney=log⁡2(−5x4) y=\log _{2}\left(-5 x^{4}\right) y=log2​(−5x4)\newlineAnswer: y′= y^{\prime}= y′=

Find the derivative of the following function. $\newline$ $y=\log _{2}\left(-5 x^{4}\right)$ $\newline$ Answer: $y^{\prime}=$