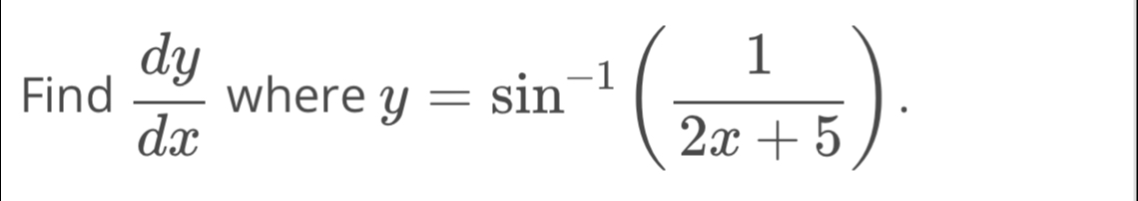Find (dy)/(dx) where y=sin^(-1)((1)/(2x+5))

Question

Find  (dy)/(dx) where  y=sin^(-1)((1)/(2x+5))

Bytelearn · Accepted Answer

Define Function: We are given the function y = sin^(-1)((1)/(2x+5)). To find the derivative dy/dx, we will use the chain rule and the derivative of the inverse sine function. The derivative of sin^(-1)(u) with respect to u is 1/sqrt(1-u^2), where u is a function of x. Let u = (1)/(2x+5), then du/dx = -2/(2x+5)^2.Apply Chain Rule: Now we apply the chain rule to find dy/dx. The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function. So, dy/dx = (d/dx)(sin^(-1)(u)) * (du/dx).Substitute Derivatives: Substitute the derivative of sin^(-1)(u) and du/dx into the chain rule expression. dy/dx = (1/sqrt(1-u^2)) * (-2/(2x+5)^2).Simplify Square Root: Now we substitute u back into the expression to get the derivative in terms of x. dy/dx = (1/sqrt(1-((1)/(2x+5))^2)) * (-2/(2x+5)^2).Write Full Expression: Simplify the expression inside the square root. 1-((1)/(2x+5))^2 = 1 - (1/(4x^2+20x+25)) = (4x^2+20x+25 - 1)/(4x^2+20x+25) = (4x^2+20x+24)/(4x^2+20x+25).Combine Terms: Now we can write the full expression for dy/dx. dy/dx = (1/sqrt((4x^2+20x+24)/(4x^2+20x+25))) * (-2/(2x+5)^2).Final Simplified Expression: Simplify the square root by combining the terms. sqrt((4x^2+20x+24)/(4x^2+20x+25)) = sqrt(1 - (1/(4x^2+20x+25))).Now we can write the final simplified expression for dy/dx. dy/dx = (-2/(2x+5)^2) / sqrt(1 - (1/(4x^2+20x+25))).

Find $\frac{d y}{d x}$ where $y=\sin ^{-1}\left(\frac{1}{2 x+5}\right)$

Full solution

More problems from Evaluate expression when two complex numbers are given

Related topics

Find dydx \frac{d y}{d x} dxdy​ where y=sin⁡−1(12x+5) y=\sin ^{-1}\left(\frac{1}{2 x+5}\right) y=sin−1(2x+51​)

Find $\frac{d y}{d x}$ where $y=\sin ^{-1}\left(\frac{1}{2 x+5}\right)$