Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Find all angles,
0^(@) <= theta < 360^(@), that satisfy the equation below, to the nearest tenth of a degree.

-4cos^(2)theta+13 cos theta-5=9cos theta-8
Answer:
theta=

Find all angles, $0^{\circ} \leq \theta<360^{\circ}$ , that satisfy the equation below, to the nearest tenth of a degree. $\newline$ $-4 \cos ^{2} \theta+13 \cos \theta-5=9 \cos \theta-8$ $\newline$ Answer: $\theta=$

View step-by-step help

View step-by-step help

Find the roots of factored polynomials

Full solution

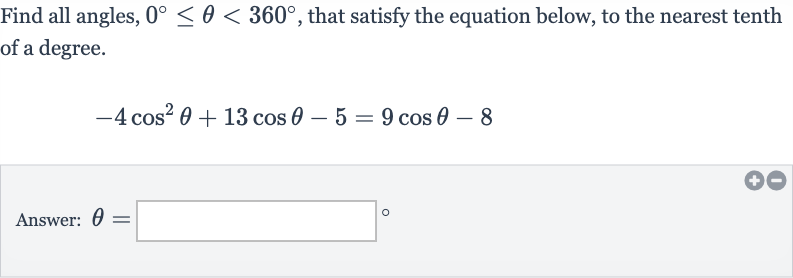
Q. Find all angles,

0^{\circ} \leq \theta<360^{\circ}

, that satisfy the equation below, to the nearest tenth of a degree.

\newline

-4 \cos ^{2} \theta+13 \cos \theta-5=9 \cos \theta-8

\newline

Answer:

\theta=

Rewrite Equation: First, let's rewrite the given equation to collect like terms on one side: $\newline$ $-4\cos^2(\theta) + 13\cos(\theta) - 5 = 9\cos(\theta) - 8$
Combine Like Terms: Now, subtract $9\cos(\theta)$ from both sides and add $8$ to both sides to get all terms involving $\cos(\theta)$ on one side and the constant on the other side: $\newline$ $-4\cos^2(\theta) + 13\cos(\theta) - 5 - 9\cos(\theta) + 8 = 0$
Quadratic Equation: Simplify the equation by combining like terms: $\newline$ $-4\cos^2(\theta) + 4\cos(\theta) + 3 = 0$
Factorize: This is a quadratic equation in terms of $\cos(\theta)$ . Let's set $u = \cos(\theta)$ to make it easier to solve: $\newline$ $-4u^2 + 4u + 3 = 0$
Solve for $u$ : Now, we can factor the quadratic equation: $\newline$ $-4u^2 + 4u + 3 = 0$ $\newline$ $(2u + 1)(-2u + 3) = 0$
Find Possible Values: Set each factor equal to zero and solve for $u$ : $2u + 1 = 0$ or $-2u + 3 = 0$
Discard Invalid Value: Solve the first equation for $u$ : $2u + 1 = 0$ $2u = -1$ $u = -\frac{1}{2}$
Find Corresponding Angles: Solve the second equation for $u$ :\(\newline\) $-2$ $u$ + $3$ = $0$ \(\newline\) $-2$ $u$ = $-3$ \(\newline\) $u$ = $\frac{3}{2}$
Final Answer: Since $u = \cos(\theta)$ , we have two possible values for $\cos(\theta)$ : $-\frac{1}{2}$ and $\frac{3}{2}$ . However, the cosine of an angle cannot be greater than $1$ , so we discard the value $\frac{3}{2}$ .
Final Answer: Since $u = \cos(\theta)$ , we have two possible values for $\cos(\theta)$ : $-\frac{1}{2}$ and $\frac{3}{2}$ . However, the cosine of an angle cannot be greater than $1$ , so we discard the value $\frac{3}{2}$ .Now we find the angles $\theta$ that correspond to $\cos(\theta) = -\frac{1}{2}$ . The cosine of $-\frac{1}{2}$ occurs at $120$ degrees and $\cos(\theta)$ $0$ degrees in the range of $\cos(\theta)$ $1$ to $\cos(\theta)$ $2$ degrees.
Final Answer: Since $u = \cos(\theta)$ , we have two possible values for $\cos(\theta)$ : $-\frac{1}{2}$ and $\frac{3}{2}$ . However, the cosine of an angle cannot be greater than $1$ , so we discard the value $\frac{3}{2}$ .Now we find the angles $\theta$ that correspond to $\cos(\theta) = -\frac{1}{2}$ . The cosine of $-\frac{1}{2}$ occurs at $120$ degrees and $\cos(\theta)$ $0$ degrees in the range of $\cos(\theta)$ $1$ to $\cos(\theta)$ $2$ degrees.We can now write the final answer with the angles that satisfy the original equation: $\newline$ $\cos(\theta)$ $3$ degrees, $\cos(\theta)$ $0$ degrees

More problems from Find the roots of factored polynomials

Question

Find the degree of this polynomial.

\newline

`5b^{10} + 3b^3`

\newline

Posted 2 months ago

Question

\newline

(9a + 5) - (2a + 4)

\newline

Posted 2 months ago

Question

Find the product. Simplify your answer.

\newline

-3v^2(v^2 - 9)

\newline

Posted 1 month ago

Question

Find the product. Simplify your answer.

\newline

(v - 3)(4v + 1)

\newline

Posted 2 months ago

Question

Find the square. Simplify your answer.

\newline

(3y + 2)^2

\newline

Posted 2 months ago

Question

Divide. If there is a remainder, include it as a simplified fraction.

\newline

(24t^2 + 36t) \div 6t

\newline

Posted 2 months ago

Question

Is the function

q(x) = x^6 - 9

even, odd, or neither?

\newline

\newline

\text{[[even][odd][neither]]}

Posted 2 months ago

Question

Find the product. Simplify your answer.

\newline

-3q^2(-3q^2 + q)

\newline

Posted 2 months ago

Question

Find the product. Simplify your answer.

\newline

(r + 3)(4r + 2)

\newline

Posted 2 months ago

Question

Find the roots of the factored polynomial.

\newline

(x + 7)(x + 4)

\newline

Write your answer as a list of values separated by commas.

\newline

x =

Posted 2 months ago