AI tutor
Full solution
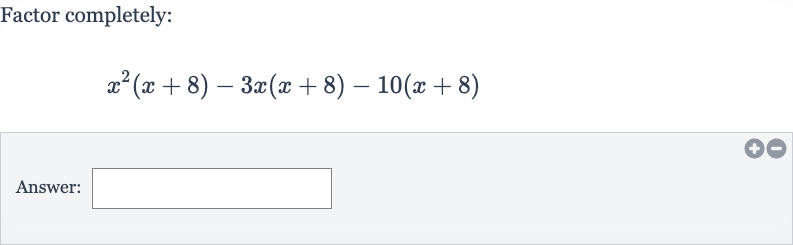
Q. Factor completely:Answer:
- Identify Common Factor: Identify the common factor in all terms.Looking at the expression , we can see that is a common factor in each term.
- Factor Out Common Factor: Factor out the common factor . We take out of each term, which gives us .
- Factor Quadratic Expression: Factor the quadratic expression.Now we need to factor the quadratic . We look for two numbers that multiply to and add up to . These numbers are and .
- Write Factored Form: Write the factored form of the quadratic.The quadratic factors into .
- Combine Factored Expressions: Combine the factored quadratic with the common factor.The completely factored form of the original expression is .
More problems from Composition of linear and quadratic functions: find a value
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help