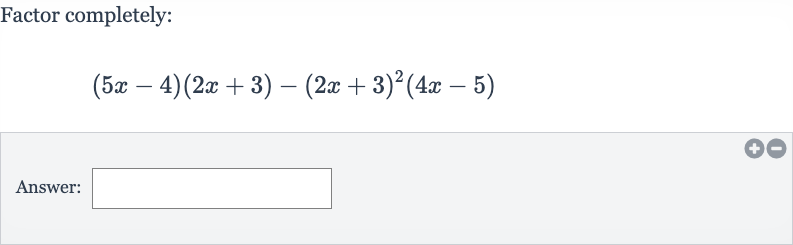Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Factor completely: (5x-4)(2x+3)-(2x+3)^(2)(4x-5) Answer:

Factor completely:\newline(5x−4)(2x+3)−(2x+3)2(4x−5) (5 x-4)(2 x+3)-(2 x+3)^{2}(4 x-5) \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Transformations of functionsright chevron icon

Full solution

Q. Factor completely:\newline(5x−4)(2x+3)−(2x+3)2(4x−5) (5 x-4)(2 x+3)-(2 x+3)^{2}(4 x-5) \newlineAnswer:
  1. Distribute Common Factor: Distribute the common factor (2x+3)(2x+3) in the expression.\newlineWe have the expression (5x−4)(2x+3)−(2x+3)2(4x−5)(5x-4)(2x+3) - (2x+3)^2(4x-5). Notice that (2x+3)(2x+3) is a common factor in both terms. We can factor it out to simplify the expression.\newlineCalculation: (2x+3)[(5x−4)−(2x+3)(4x−5)](2x+3)[(5x-4) - (2x+3)(4x-5)]
  2. Expand Squared Term: Expand the squared term and distribute the negative sign.\newlineWe need to expand (2x+3)2(2x+3)^2 and then distribute the negative sign through the resulting expression.\newlineCalculation: (2x+3)2=(2x+3)(2x+3)=4x2+6x+6x+9=4x2+12x+9(2x+3)^2 = (2x+3)(2x+3) = 4x^2 + 6x + 6x + 9 = 4x^2 + 12x + 9\newlineNow distribute the negative sign: −(4x2+12x+9)=−4x2−12x−9-(4x^2 + 12x + 9) = -4x^2 - 12x - 9
  3. Substitute Expanded Term: Substitute the expanded squared term back into the expression.\newlineNow we substitute the expanded and negated squared term back into the expression we obtained after factoring out (2x+3)(2x+3).\newlineCalculation: (2x+3)[(5x−4)−(4x2+12x+9)(4x−5)](2x+3)[(5x-4) - (4x^2 + 12x + 9)(4x-5)]
  4. Distribute Quadratic Term: Distribute (4x−5)(4x-5) through the quadratic term.\newlineWe need to distribute (4x−5)(4x-5) through the quadratic term (4x2+12x+9)(4x^2 + 12x + 9).\newlineCalculation: (4x−5)(4x2+12x+9)=4x(4x2+12x+9)−5(4x2+12x+9)((4x-5)(4x^2 + 12x + 9) = 4x(4x^2 + 12x + 9) - 5(4x^2 + 12x + 9)(\newline= 16x^3 + 48x^2 + 36x - 20x^2 - 60x - 45(\newline\)= 16x^3 + 28x^2 - 24x - 45\)
  5. Combine Distributed Terms: Combine the distributed terms with the remaining term inside the brackets.\newlineNow we combine the result from the previous step with the remaining term (5x−4)(5x-4) inside the brackets.\newlineCalculation: (2x+3)[(5x-4) - (16x^3 + 28x^2 - 24x - 45)]\(\newline= (2x+3)(5x - 4 - 16x^3 - 28x^2 + 24x + 45)\)
  6. Combine Like Terms: Combine like terms inside the brackets.\newlineWe need to combine like terms inside the brackets to simplify the expression further.\newlineCalculation: (2x+3)(−16x3−28x2+(5x+24x)−4+45)(2x+3)(-16x^3 - 28x^2 + (5x + 24x) - 4 + 45)\newline= (2x+3)(−16x3−28x2+29x+41)(2x+3)(-16x^3 - 28x^2 + 29x + 41)
  7. Factor Out Common Factor: Factor out the common factor (2x+3)(2x+3) completely.\newlineWe have already factored out the common factor (2x+3)(2x+3) in Step 11, and now we have the expression simplified inside the brackets. The expression is now completely factored.\newlineCalculation: (2x+3)(−16x3−28x2+29x+41)(2x+3)(-16x^3 - 28x^2 + 29x + 41)

More problems from Transformations of functions

Question
Find g(x)g(x), where g(x)g(x) is the reflection across the x-axis of f(x)=9∣x+2∣−4f(x)=9|x+2|-4.\newlineChoices:\newline(A)g(x)=9∣x+2∣−4]\text{}(A)g(x) = 9|x+2| - 4\text{]}\newline(B)g(x)=9∣x−2∣−4]\text{}(B)g(x) = 9|x-2| - 4\text{]}\newline(C)g(x)=−9∣x−2∣+4]\text{}(C)g(x)=-9|x-2| +4\text{]}\newline(D)g(x)=−9∣x+2∣+4]\text{(D)}g(x) = -9|x + 2| + 4\text{]}
Get tutor helpright-arrow

Posted 9 months ago

Question
What does the transformation f(x)↦f(x−4) f(x) \mapsto f(x - 4) do to the graph of f(x) f(x) ?\newlineChoices:\newline(A) translates it 4 units right\text{translates it } 4 \text{ units right}\newline(B) translates it 4 units down\text{translates it } 4 \text{ units down}\newline(C) translates it 4 units left\text{translates it } 4 \text{ units left}\newline(D) translates it 4 units up\text{translates it } 4 \text{ units up}
Get tutor helpright-arrow

Posted 9 months ago

Question
What does the transformation f(x)↦f(4x) f(x) \mapsto f(4x) do to the graph of f(x) f(x) ?\newlineChoices:\newline[A] stretches it vertically\newline[B] stretches it horizontally\newline[C]shrinks it horizontally\newline[D]shrinks it vertically
Get tutor helpright-arrow

Posted 8 months ago

Question
Find g(x)g(x), where g(x)g(x) is the translation 99 units down of f(x)=10x+8f(x) = 10x + 8.\newlineChoices:\newline(A) g(x)=10x−82g(x) = 10x - 82 \newline(B) g(x)=10x+98g(x) = 10x + 98 \newline(C) g(x)=10x−1g(x) = 10x - 1\newline(D) g(x)=10x+17g(x) = 10x + 17
Get tutor helpright-arrow

Posted 9 months ago

Question
What kind of transformation converts the graph of f(x)=−3x2−4 f(x) = -3x^2 - 4 into the graph of g(x)=−3x2+6 g(x) = -3x^2 + 6 ?\newlineChoices:\newline[A]translation 10 units up \text{[A]translation 10 units up} \newline[B]translation 10 units down \text{[B]translation 10 units down} \newline[C]translation 10 units left \text{[C]translation 10 units left} \newline[D]translation 10 units right \text{[D]translation 10 units right}
Get tutor helpright-arrow

Posted 8 months ago

Question
The parabola y=x2y=x^{2} is scaled vertically by a factor of 77. What is the equation of the new parabola?\newliney=y=
Get tutor helpright-arrow

Posted 10 months ago

Question
The parabola y=x2y=x^{2} is shifted down by 66 units and to the right by 55 units.\newlineWhat is the equation of the new parabola?\newliney=y=
Get tutor helpright-arrow

Posted 10 months ago

Question
The parabola y=x2y=x^{2} is shifted up by 22 units and to the right by 33 units.\newlineWhat is the equation of the new parabola?\newliney=y=
Get tutor helpright-arrow

Posted 8 months ago

Question
The parabola y=x2 y=x^{2} is reflected across the x x -axis and then scaled vertically by a factor of 55 .\newlineWhat is the equation of the new parabola?\newliney=□ y=\square
Get tutor helpright-arrow

Posted 8 months ago

Question
The parabola y=x2 y=x^{2} is scaled vertically by a factor of 110 \frac{1}{10} .\newlineWhat is the equation of the new parabola?\newliney= y=
Get tutor helpright-arrow

Posted 9 months ago

View all (82)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant