Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Factor completely: 25x^(2)(x-5)-(x-5) Answer:

Factor completely:\newline25x2(x−5)−(x−5) 25 x^{2}(x-5)-(x-5) \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Grade 8right chevron icon
Estimate positive square rootsright chevron icon

Full solution

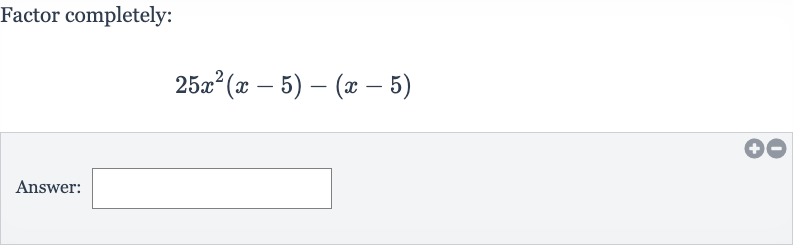
Q. Factor completely:\newline25x2(x−5)−(x−5) 25 x^{2}(x-5)-(x-5) \newlineAnswer:
  1. Identify Common Factor: Identify the common factor in both terms.\newlineThe common factor in both terms is (x−5)(x-5).
  2. Factor Out Common Factor: Factor out the common factor (x−5)(x-5).\newlineThe expression can be rewritten as (x−5)(25x2−1)(x-5)(25x^2 - 1).
  3. Recognize Difference of Squares: Recognize that the second term is a difference of squares. 25x2−125x^2 - 1 can be factored as (5x+1)(5x−1)(5x + 1)(5x - 1) because it is a difference of squares where a2−b2=(a+b)(a−b)a^2 - b^2 = (a + b)(a - b).
  4. Write Final Factored Form: Write the final factored form.\newlineThe complete factorization of the expression is (x−5)(5x+1)(5x−1)(x-5)(5x + 1)(5x - 1).

More problems from Estimate positive square roots

Question
What is 49 \sqrt{49} ??\newline____
Get tutor helpright-arrow

Posted 1 month ago

Question
Complete the following statement. Use the integers that are closest to the number in the middle.\newline____ <<40\sqrt{40} << ___
Get tutor helpright-arrow

Posted 1 month ago

Question
What is −64-\sqrt{64}?\newline____
Get tutor helpright-arrow

Posted 2 months ago

Question
What is 273 \sqrt[3]{27} ?
Get tutor helpright-arrow

Posted 1 month ago

Question
Which two integers is −48-\sqrt{48} between?\newlineChoices:\newline[A]−6 and −5[A]-6 \text{ and } -5\newline[B]5 and 6[B]5 \text{ and } 6\newline[C]−7 and −6[C]-7 \text{ and } -6\newline[D]6 and 7[D]6 \text{ and } 7
Get tutor helpright-arrow

Posted 6 days ago

Question
What is the cube root of −64-64?\newline____
Get tutor helpright-arrow

Posted 2 months ago

Question
Which two integers is 223\sqrt[3]{22} between?\newlineChoices:\newline(A) [1 and 2] [1 \text{ and } 2] \newline(B) [15 and 16][15 \text{ and } 16] \newline(C) [16 and 17][16 \text{ and } 17] \newline(D) [2 and 3][2 \text{ and } 3]
Get tutor helpright-arrow

Posted 2 months ago

Question
Simplify:\newline(25)2=_____(\sqrt{25})^2 = \_\_\_\_\_
Get tutor helpright-arrow

Posted 1 month ago

Question
Look at this equation:\newlinec3=27c^3 = 27\newline\newlineWhat is cc, the cube root of 2727?\newlinec=c = ____\newline
Get tutor helpright-arrow

Posted 2 months ago

Question
Write the sentence as an equation. \newline172172 is equal to the quotient of xx and 44 \newlineType a slash ( / / ) if you want to use a division sign. \newline_____
Get tutor helpright-arrow

Posted 1 month ago

View all (35)