Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Expand the logarithm fully using the properties of logs. Express the final answer in terms of
log x,log y, and
log z.

log ((root(3)(x))/(zy^(2)))
Answer:

Expand the logarithm fully using the properties of logs. Express the final answer in terms of $\log x, \log y$ , and $\log z$ . $\newline$ $\log \frac{\sqrt[3]{x}}{z y^{2}}$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Quotient property of logarithms

Full solution

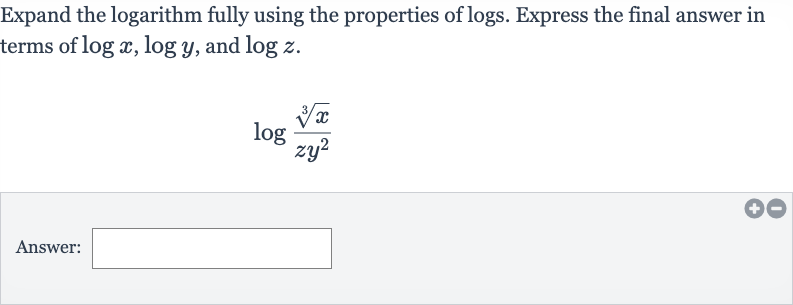
Q. Expand the logarithm fully using the properties of logs. Express the final answer in terms of

\log x, \log y

, and

\log z

.

\newline

\log \frac{\sqrt[3]{x}}{z y^{2}}

\newline

Answer:

Apply Quotient Rule: Apply the quotient rule of logarithms to the expression $\log\left(\frac{\sqrt[3]{x}}{zy^{2}}\right)$ . Quotient rule of logarithm: $\log\left(\frac{a}{b}\right) = \log(a) - \log(b)$ $\log\left(\frac{\sqrt[3]{x}}{zy^{2}}\right) = \log(\sqrt[3]{x}) - \log(zy^{2})$
Apply Cube Root Property: Apply the cube root property to the logarithm $\log(\sqrt[3]{x})$ . $\newline$ Cube root property: $\log(\sqrt[3]{a}) = \frac{1}{3} \cdot \log(a)$ $\newline$ $\log(\sqrt[3]{x}) = \frac{1}{3} \cdot \log(x)$
Apply Product Rule: Apply the product rule of logarithms to the expression $\log(zy^{2})$ . $\newline$ Product rule of logarithm: $\log(a \cdot b) = \log(a) + \log(b)$ $\newline$ $\log(zy^{2}) = \log(z) + \log(y^{2})$
Apply Power Rule: Apply the power rule to the logarithm $\log(y^{2})$ . $\newline$ Power rule of logarithm: $\log(a^{b}) = b \times \log(a)$ $\newline$ $\log(y^{2}) = 2 \times \log(y)$
Combine and Distribute: Combine the results from Steps $1$ to $4$ to get the final expanded form. $\newline$ $\log\left(\frac{\sqrt[3]{x}}{zy^{2}}\right) = \frac{1}{3} \cdot \log(x) - (\log(z) + 2 \cdot \log(y))$ $\newline$ Distribute the negative sign: $\newline$ $\log\left(\frac{\sqrt[3]{x}}{zy^{2}}\right) = \frac{1}{3} \cdot \log(x) - \log(z) - 2 \cdot \log(y)$

More problems from Quotient property of logarithms

Question

Solve. Write your answer as an integer or a fraction in simplest form.

\newline

5^x=1

\newline

x=

Posted 3 months ago

Question

Steven opened a savings account and deposited

\$100.00

as principal. The account earns

9\%

interest, compounded annually. What is the balance after

5

\newline

Use the formula

A = P(1 + \frac{r}{n})^{nt}

A

is the balance (final amount),

P

is the principal (starting amount),

r

is the interest rate expressed as a decimal,

n

is the number of times per year that the interest is compounded, and

t

is the time in years.

\newline

Round your answer to the nearest cent.

\newline

\$

Posted 3 months ago

Question

Use the following function rule to find

f(1)

\newline

f(x) = 12(7)^x + 8

\newline

f(1) =

Posted 2 months ago

Question

The town of Valley View conducted a census this year, which showed that it has a population of

1,800

people. Based on the census data, it is estimated that the population of Valley View will grow by

12\%

\newline

Write an exponential equation in the form

y = a(b)^x

that can model the town population,

y

x

decades after this census was taken.

\newline

Use whole numbers, decimals, or simplified fractions for the values of

a

b

\newline

y =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = 9^x

\newline

x =

Posted 3 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = e^x

\newline

x =

Posted 1 month ago

Question

Solve. Simplify your answer.

\newline

\log u = 1

\newline

u =

Posted 3 months ago

Question

Solve. Simplify your answer.

\newline

7\log x = 7

\newline

x =

Posted 2 months ago

Question

g(t) = 3^t

change over the interval from

t = 3

t = 4

\newline

\newline

g(t)

3

\newline

g(t)

3

\newline

g(t)

3\%

\newline

g(t)

200\%

Posted 3 months ago

Question

f(x)

6

over every unit interval in

x

f(0) = 0

\newline

Which could be a function rule for

f(x)

\newline

\newline

f(x) = 6^x

\newline

f(x) = 6x

\newline

f(x) = \frac{1}{6^x}

\newline

f(x) = \frac{x}{6}

Posted 3 months ago