Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Expand the logarithm fully using the properties of logs. Express the final answer in terms of
log x.

log 5x^(2)
Answer:

Expand the logarithm fully using the properties of logs. Express the final answer in terms of $\log x$ . $\newline$ $\log 5 x^{2}$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Product property of logarithms

Full solution

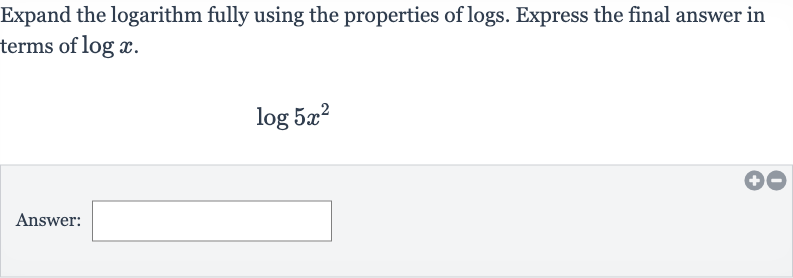
Q. Expand the logarithm fully using the properties of logs. Express the final answer in terms of

\log x

.

\newline

\log 5 x^{2}

\newline

Answer:

Identify Properties: Identify the properties of logarithms to be used for expanding $\log 5x^{2}$ . The expression $5x^{2}$ is a product of $5$ and $x^{2}$ . To expand the logarithm, we will use the product property of logarithms, which states that the logarithm of a product is equal to the sum of the logarithms of the individual factors. Additionally, we will use the power property of logarithms, which states that the logarithm of a power is equal to the exponent times the logarithm of the base.
Apply Product Property: Apply the product property to expand $\log 5x^{2}$ . Using the product property, we can write $\log 5x^{2}$ as the sum of $\log 5$ and $\log x^{2}$ . $\log 5x^{2} = \log 5 + \log x^{2}$
Apply Power Property: Apply the power property to the term $\log x^{2}$ . $\newline$ Using the power property, we can move the exponent in $\log x^{2}$ to the front of the logarithm. $\newline$ $\log x^{2} = 2 \times \log x$
Substitute Expanded Log: Substitute the expanded log $x^{2}$ back into the equation from Step $2$ . $\newline$ Now we replace $\log x^{2}$ with $2 \cdot \log x$ in the equation we obtained in Step $2$ . $\newline$ $\log 5x^{2} = \log 5 + 2 \cdot \log x$

More problems from Product property of logarithms

Question

Solve. Write your answer as an integer or a fraction in simplest form.

\newline

5^x=1

\newline

x=

Posted 2 months ago

Question

Steven opened a savings account and deposited

\$100.00

as principal. The account earns

9\%

interest, compounded annually. What is the balance after

5

\newline

Use the formula

A = P(1 + \frac{r}{n})^{nt}

A

is the balance (final amount),

P

is the principal (starting amount),

r

is the interest rate expressed as a decimal,

n

is the number of times per year that the interest is compounded, and

t

is the time in years.

\newline

Round your answer to the nearest cent.

\newline

\$

Posted 2 months ago

Question

Use the following function rule to find

f(1)

\newline

f(x) = 12(7)^x + 8

\newline

f(1) =

Posted 1 month ago

Question

The town of Valley View conducted a census this year, which showed that it has a population of

1,800

people. Based on the census data, it is estimated that the population of Valley View will grow by

12\%

\newline

Write an exponential equation in the form

y = a(b)^x

that can model the town population,

y

x

decades after this census was taken.

\newline

Use whole numbers, decimals, or simplified fractions for the values of

a

b

\newline

y =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = 9^x

\newline

x =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = e^x

\newline

x =

Posted 1 month ago

Question

Solve. Simplify your answer.

\newline

\log u = 1

\newline

u =

Posted 2 months ago

Question

Solve. Simplify your answer.

\newline

7\log x = 7

\newline

x =

Posted 1 month ago

Question

g(t) = 3^t

change over the interval from

t = 3

t = 4

\newline

\newline

g(t)

3

\newline

g(t)

3

\newline

g(t)

3\%

\newline

g(t)

200\%

Posted 2 months ago

Question

f(x)

6

over every unit interval in

x

f(0) = 0

\newline

Which could be a function rule for

f(x)

\newline

\newline

f(x) = 6^x

\newline

f(x) = 6x

\newline

f(x) = \frac{1}{6^x}

\newline

f(x) = \frac{x}{6}

Posted 2 months ago