AI tutor
Full solution
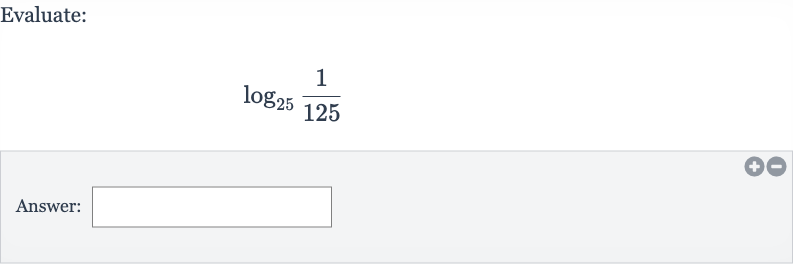
Q. Evaluate:Answer:
- Understand Problem: Understand the problem and identify the properties of logarithms to use.We need to evaluate the logarithm of with base . We can use the change of base formula for logarithms, which is , where all the logarithms are in the same base.
- Apply Change of Base: Apply the change of base formula.Using the change of base formula, we can write the expression as:We know that is log base of , and is log base of /.
- Evaluate Logarithms: Evaluate the logarithms.Since is squared and is cubed, we can rewrite the logarithms as:
- Simplify Expression: Simplify the expression.Now we can divide the two expressions: = This simplifies to: =
- Check for Errors: Check for any mathematical errors. We have used the properties of logarithms correctly and simplified the expression without any mistakes.