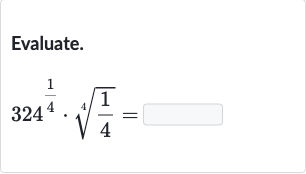Evaluate. 324^((1)/(4))*root(4)((1)/(4))=

Question

Evaluate.  324^((1)/(4))*root(4)((1)/(4))=

Bytelearn · Accepted Answer

Problem Understanding: Understand the problem. We need to find the value of the fourth root of 324 multiplied by the fourth root of 1/4. Calculate Fourth Root of 324: Calculate the fourth root of 324. The fourth root of 324 is the number that when raised to the power of 4 gives 324. We can find this number by recognizing that 324 is a perfect square and a perfect fourth power, as 18^2 = 324 and 3^4 = 81. Therefore, the fourth root of 324 is 3^2, which is 9. Calculate Fourth Root of 1/4: Calculate the fourth root of 1/4. The fourth root of 1/4 is the number that when raised to the power of 4 gives 1/4. We know that (1/2)^4 = 1/16, so the fourth root of 1/4 must be slightly larger than 1/2. Since (1/2)^2 = 1/4, the fourth root of 1/4 is 1/2. Multiply Results: Multiply the results from Step 2 and Step 3. Now we multiply the fourth root of 324, which is 9, by the fourth root of 1/4, which is 1/2. So, 9 * (1/2) = 9/2 = 4.5.

Evaluate. $\newline$ $324^{\frac{1}{4}} \cdot \sqrt[4]{\frac{1}{4}}=$

Full solution

More problems from Multiply two decimals: where does the decimal point go?

Related topics

Evaluate.\newline32414⋅144= 324^{\frac{1}{4}} \cdot \sqrt[4]{\frac{1}{4}}= 32441​⋅441​​=

Evaluate. $\newline$ $324^{\frac{1}{4}} \cdot \sqrt[4]{\frac{1}{4}}=$