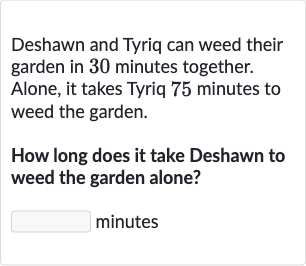
Deshawn and Tyriq can weed their garden in 30 minutes together. Alone, it takes Tyriq 75 minutes to weed the garden. How long does it take Deshawn to weed the garden alone? minutes

Question

Bytelearn · Accepted Answer

Define Rates: Let's denote the rate at which Deshawn can weed the garden alone as D (gardens per minute) and the rate at which Tyriq can weed the garden alone as T (gardens per minute). We know that Tyriq can weed the garden in 75 minutes, so his rate is T = 1 garden / 75 minutes.Express Combined Rate: Since Deshawn and Tyriq can weed the garden together in 30 minutes, their combined rate is 1 garden / 30 minutes. We can express this combined rate as D + T.Set Up Equation: Now we can set up the equation for their combined rate: D + T = 1/30. We already know that T = 1/75, so we can substitute this into the equation to find D.Substitute and Solve: Substituting T into the equation gives us D + 1/75 = 1/30. To solve for D, we subtract 1/75 from both sides of the equation.Find Common Denominator: After subtracting, we get D = 1/30 - 1/75. To subtract these fractions, we need a common denominator, which is 150.Convert Fractions: Converting both fractions to have a denominator of 150 gives us D = (5/150) - (2/150).Subtract Fractions: Subtracting the fractions gives us D = (5 - 2) / 150, which simplifies to D = 3/150.Simplify Fraction: Simplifying the fraction 3/150 gives us D = 1/50. This means Deshawn can weed 1 garden in 50 minutes alone.

Deshawn and Tyriq can weed their garden in $30$ minutes together. Alone, it takes Tyriq $75$ minutes to weed the garden. $\newline$ How long does it take Deshawn to weed the garden alone? $\newline$ minutes

Full solution

More problems from Solve proportions: word problems

Related topics

Deshawn and Tyriq can weed their garden in 303030 minutes together. Alone, it takes Tyriq 757575 minutes to weed the garden.\newlineHow long does it take Deshawn to weed the garden alone?\newlineminutes

Deshawn and Tyriq can weed their garden in $30$ minutes together. Alone, it takes Tyriq $75$ minutes to weed the garden. $\newline$ How long does it take Deshawn to weed the garden alone? $\newline$ minutes