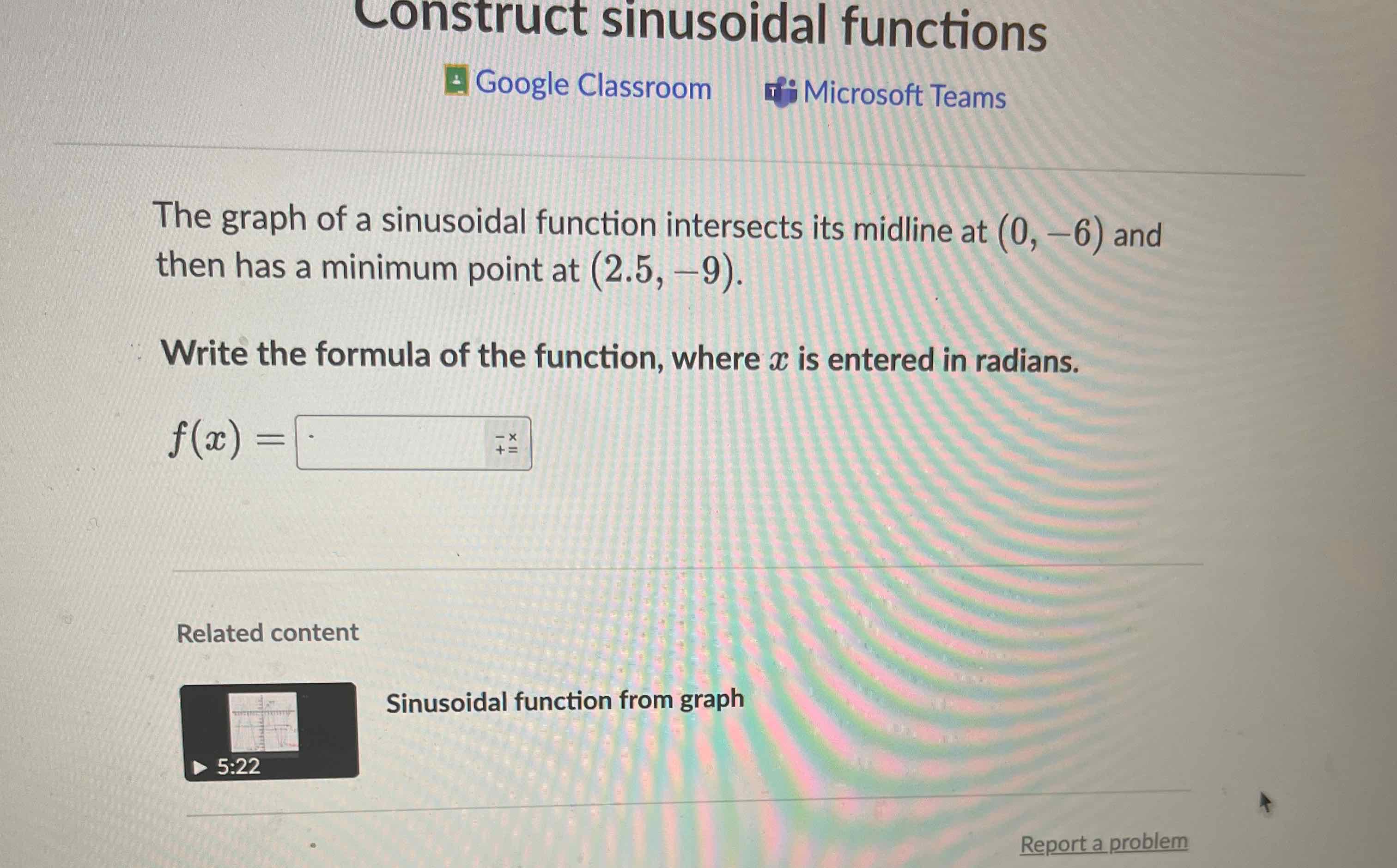
The graph of a sinusoidal function intersects its midline at (0,-6) and then has a minimum point at (2.5,-9). Write the formula of the function, where x is entered in radians. f(x)= ◻

Question

The graph of a sinusoidal function intersects its midline at  (0,-6) and then has a minimum point at  (2.5,-9). Write the formula of the function, where  x is entered in radians.  f(x)=  ◻

Bytelearn · Accepted Answer

Determine Midline and Amplitude:  Determine the midline and amplitude of the sinusoidal function. The midline is given by the y-coordinate of the intersection with the midline, which is -6. The minimum point is at (2.5, -9). The amplitude is the distance from the midline to the minimum or maximum point. Here, the distance from -6 to -9 is 3, so the amplitude is 3. Identify Period and Phase Shift:  Identify the period and phase shift. Since the function reaches a minimum at x = 2.5, and this is the first minimum after crossing the midline at x = 0, we can determine that the period is 4 times 2.5, which is 10. There is no horizontal shift since the sinusoidal function crosses the midline at x = 0. Write Sinusoidal Function:  Write the sinusoidal function formula. We know the function has a minimum at (2.5, -9) and crosses the midline at (0, -6). Since it's a minimum, we use a negative cosine function. The formula is: f(x) = -3cos((π/5)x) - 6

The graph of a sinusoidal function intersects its midline at $(0,-6)$ and then has a minimum point at $(2.5,-9)$ . $\newline$ Write the formula of the function, where $x$ is entered in radians. $\newline$ $f(x)=$ $\square$

Full solution

More problems from Conjugate root theorems

Related topics

The graph of a sinusoidal function intersects its midline at (0,−6) (0,-6) (0,−6) and then has a minimum point at (2.5,−9) (2.5,-9) (2.5,−9).\newlineWrite the formula of the function, where x x x is entered in radians.\newlinef(x)= f(x)= f(x)= □ \square □

The graph of a sinusoidal function intersects its midline at $(0,-6)$ and then has a minimum point at $(2.5,-9)$ . $\newline$ Write the formula of the function, where $x$ is entered in radians. $\newline$ $f(x)=$ $\square$