Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Consider the curve given by the equation
xy^(2)+5xy=50. It can be shown that

(dy)/(dx)=(-y(y+5))/(x(2y+5))". "
Write the equation of the vertical line that is tangent to the curve.

◻

Consider the curve given by the equation $\newline$ $xy^{2}+5xy=50$ . It can be shown that $\newline$ $(dy)/(dx)=(-y(y+5))/(x(2y+5))$ . $\newline$ Write the equation of the vertical line that is tangent to the curve.

View step-by-step help

View step-by-step help

Find equations of tangent lines using limits

Full solution

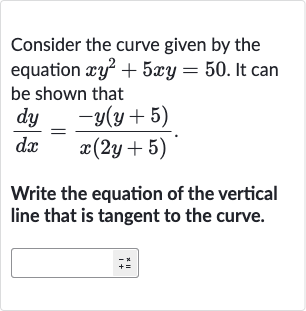
Q. Consider the curve given by the equation

\newline

xy^{2}+5xy=50

. It can be shown that

\newline

(dy)/(dx)=(-y(y+5))/(x(2y+5))

.

\newline

Write the equation of the vertical line that is tangent to the curve.

Set Denominator Equal to Zero: To find the vertical tangent, we need to set the denominator of the derivative equal to $0$ because the slope of a vertical line is undefined, which corresponds to an infinite slope or a $0$ in the denominator of the derivative.
Solve for $x$ : Set the denominator of $\frac{dy}{dx}$ equal to zero: $x(2y + 5) = 0$ .
Find x-coordinate: Solve for $x$ to find the $x$ -coordinate where the vertical tangent occurs: $x = 0$ .
Equation of Vertical Line: The equation of a vertical line is of the form $x = a$ , where $a$ is the $x$ -coordinate of any point on the line.
Equation of Vertical Line: The equation of a vertical line is of the form $x = a$ , where $a$ is the $x$ -coordinate of any point on the line.Therefore, the equation of the vertical line that is tangent to the curve is $x = 0$ .

More problems from Find equations of tangent lines using limits

Question

Consider the curve given by the equation

x y^{2}+5 x y=50

. It can be shown that

\frac{d y}{d x}=\frac{-y(y+5)}{x(2 y+5)} \text {. }

\newline

Write the equation of the vertical line that is tangent to the curve.

Posted 1 month ago

Question

The rate of change

\frac{d P}{d t}

of the number of algae in a tank is modeled by the following differential equation:

\newline

\frac{d P}{d t}=\frac{2317}{10614} P\left(1-\frac{P}{662}\right)

\newline

t=0

, the number of algae in the tank is

174

and is increasing at a rate of

28

algae per minute. At what value of

P

P(t)

growing the fastest?

\newline

Posted 2 months ago

Question

The rate of change

\frac{d P}{d t}

of the number of bacteria in a tank is modeled by the following differential equation:

\newline

\frac{d P}{d t}=\frac{2}{9849} P(598-P)

\newline

t=0

, the number of bacteria in the tank is

196

and is increasing at a rate of

16

bacteria per minute. At what value of

P

does the graph of

P(t)

have an inflection point?

\newline

Posted 2 months ago

Question

The rate of change

\frac{d P}{d t}

of the number of people infected by a disease is modeled by the following differential equation:

\newline

\frac{d P}{d t}=\frac{45}{125404} P(800-P)

\newline

t=0

, the number of people infected by the disease is

214

and is increasing at a rate of

45

people per hour. What is the limiting value for the total number of people infected by the disease as time increases?

\newline

Posted 2 months ago

Question

Which of the following is a correct interpretation of the expression

\newline

-4+13

\newline

1

\newline

(A) The number that is

4

-13

on the number line

\newline

(B) The number that is

4

to the right of

-13

on the number line

\newline

(C) The number that is

13

-4

on the number line

\newline

(D) The number that is

13

to the right of

-4

on the number line

Posted 2 months ago

Question

f

f(x)=x^{3}+2 \sin (3 x-3)

. Use a calculator to write the equation of the line tangent to the graph of

f

x=0.5

. You should round all decimals to

3

\newline

Posted 2 months ago

Question

f

f(x)=x^{2}-2 x+3 \cos \left(x^{2}-x\right)

. Use a calculator to write the equation of the line tangent to the graph of

f

x=-2.5

. You should round all decimals to

3

\newline

Posted 2 months ago

Question

f

f(x)=x^{2}+x-2 \sin (2 x)

. Use a calculator to write the equation of the line tangent to the graph of

f

x=3

. You should round all decimals to

3

\newline

Posted 2 months ago

Question

f

f(x)=x^{3}+\cos \left(2 x^{2}+5\right)

. Use a calculator to write the equation of the line tangent to the graph of

f

x=1

. You should round all decimals to

3

\newline

Posted 2 months ago

Question

f

f(x)=x^{3}+3-5 \sin \left(x^{2}\right)

. Use a calculator to write the equation of the line tangent to the graph of

f

x=1

. You should round all decimals to

3

\newline

Posted 2 months ago