AI tutor
Full solution
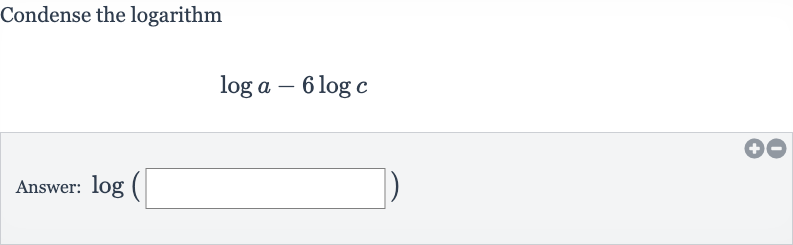
Q. Condense the logarithmAnswer:
- Identify Property: Identify the property used to condense the logarithmic expression.The expression involves a difference of logarithms, where one of the terms is a multiple of a logarithm. To condense this expression, we use the power property of logarithms, which states that .
- Apply Power Property: Apply the power property to the term with the multiple.The power property allows us to rewrite as . This is because multiplying a logarithm by a number is equivalent to raising the argument of the logarithm to the power of that number.
- Combine Using Quotient Property: Combine the two logarithms into a single logarithm using the quotient property.The quotient property of logarithms states that . We can apply this property to combine and into a single logarithm.
- Write Final Expression: Write the final condensed logarithmic expression.Using the quotient property, we combine the two logarithms into one: .
More problems from Quotient property of logarithms
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help