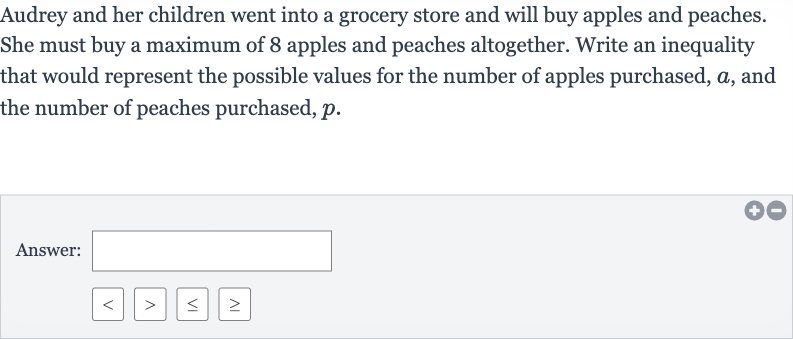
Audrey and her children went into a grocery store and will buy apples and peaches. She must buy a maximum of 8 apples and peaches altogether. Write an inequality that would represent the possible values for the number of apples purchased, a, and the number of peaches purchased, p. Answer:

Question

Audrey and her children went into a grocery store and will buy apples and peaches. She must buy a maximum of 8 apples and peaches altogether. Write an inequality that would represent the possible values for the number of apples purchased,  a, and the number of peaches purchased,  p. Answer:

Bytelearn · Accepted Answer

Define Variables: Let's define the variables: a = number of apples purchased p = number of peaches purchased The problem states that Audrey can buy a maximum of 8 apples and peaches altogether.Write Inequality: We need to write an inequality that represents the sum of apples and peaches being less than or equal to 8. The inequality will be: a + p ≤ 8Check Inequality: Now, let's check if the inequality makes sense in the context of the problem. If Audrey buys 8 apples and no peaches, the inequality would be 8 + 0 ≤ 8, which is true. If she buys 5 apples and 3 peaches, the inequality would be 5 + 3 ≤ 8, which is also true. If she buys 9 apples and no peaches, the inequality would be 9 + 0 ≤ 8, which is false, and that's consistent with the problem's condition that she cannot buy more than 8 fruits in total.

Full solution

More problems from One-step inequalities: word problems

Related topics