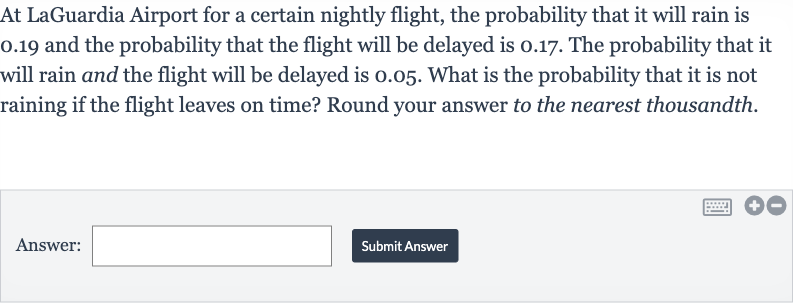
At LaGuardia Airport for a certain nightly flight, the probability that it will rain is 0.19 and the probability that the flight will be delayed is 0.17 . The probability that it will rain and the flight will be delayed is 0.05 . What is the probability that it is not raining if the flight leaves on time? Round your answer to the nearest thousandth. Answer: Submit Answer

Question

Bytelearn · Accepted Answer

Denote Events: Let's denote the events as follows: R: It will rain. D: The flight will be delayed. We are given the following probabilities: P(R) = 0.19 P(D) = 0.17 P(R and D) = 0.05  We want to find the probability that it is not raining given that the flight leaves on time. This is a conditional probability problem, and we can denote the event of the flight leaving on time as D'. The probability we are looking for is P(R' | D'), where R' is the complement of R (it does not rain).Find P(D'): First, we need to find the probability of the flight leaving on time, which is the complement of the flight being delayed. This is given by: P(D') = 1 - P(D) Calculating this, we get: P(D') = 1 - 0.17 = 0.83Find P(R and D'): Next, we need to find the probability of it not raining and the flight leaving on time, which is P(R' and D'). We can use the fact that P(R and D') is the complement of P(R and D) within the event D'. So we have: P(R and D') = P(D') - P(R and D) Calculating this, we get: P(R and D') = 0.83 - 0.05 = 0.78Calculate P(R' | D'): Now, we can find the conditional probability of it not raining given that the flight leaves on time using the formula: P(R' | D') = P(R' and D') / P(D') However, we need to adjust our previous step because we calculated P(R and D'), not P(R' and D'). We need to find P(R' and D') which is the probability of it not raining and the flight leaving on time. This is given by: P(R' and D') = P(D') - P(R and D') But we already have P(D'), so we need to find P(R') first, which is the complement of P(R): P(R') = 1 - P(R) Calculating this, we get: P(R') = 1 - 0.19 = 0.81Adjust Previous Step: Now we can correctly calculate P(R' and D') as: P(R' and D') = P(R') * P(D') This is because R' and D' are independent events, and the probability of both occurring is the product of their individual probabilities. Calculating this, we get: P(R' and D') = 0.81 * 0.83 P(R' and D') = 0.6723Calculate P(R' and D'): Finally, we can calculate the conditional probability P(R' | D') using the correct values: P(R' | D') = P(R' and D') / P(D') Substituting the values we have: P(R' | D') = 0.6723 / 0.83 Calculating this, we get: P(R' | D') ≈ 0.810

Full solution

More problems from Find probabilities using the addition rule

Related topics