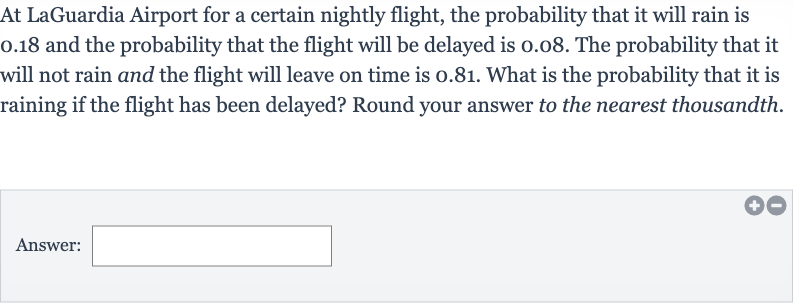
At LaGuardia Airport for a certain nightly flight, the probability that it will rain is 0.18 and the probability that the flight will be delayed is 0.08 . The probability that it will not rain and the flight will leave on time is 0.81 . What is the probability that it is raining if the flight has been delayed? Round your answer to the nearest thousandth. Answer:

Question

Bytelearn · Accepted Answer

Events Denoted: Let's denote the events as follows: R: It will rain. D: The flight will be delayed. We are given the following probabilities: P(R) = 0.18 P(D) = 0.08 P(not R and not D) = 0.81  We need to find the probability that it is raining given that the flight has been delayed, which is P(R|D). To find this, we can use Bayes' theorem or the definition of conditional probability, which is P(R|D) = P(R and D) / P(D). However, we are not directly given P(R and D), but we can find it by using the complement of the given probability of P(not R and not D).  First, let's find P(R or D), which is the complement of P(not R and not D). Using the formula for the complement of a probability, we have: P(R or D) = 1 - P(not R and not D) P(R or D) = 1 - 0.81 P(R or D) = 0.19Find P(R or D): Now, we can use the Addition Rule of Probability to find P(R and D). The Addition Rule states that: P(R or D) = P(R) + P(D) - P(R and D)  We can rearrange this formula to solve for P(R and D): P(R and D) = P(R) + P(D) - P(R or D)  Substituting the values we have: P(R and D) = 0.18 + 0.08 - 0.19 P(R and D) = 0.07Find P(R and D): Now that we have P(R and D), we can find the conditional probability P(R|D) using the formula: P(R|D) = P(R and D) / P(D)  Substituting the values we have: P(R|D) = 0.07 / 0.08 P(R|D) = 0.875  However, we need to round our answer to the nearest thousandth, so: P(R|D) ≈ 0.875

Full solution

More problems from Find probabilities using the addition rule

Related topics