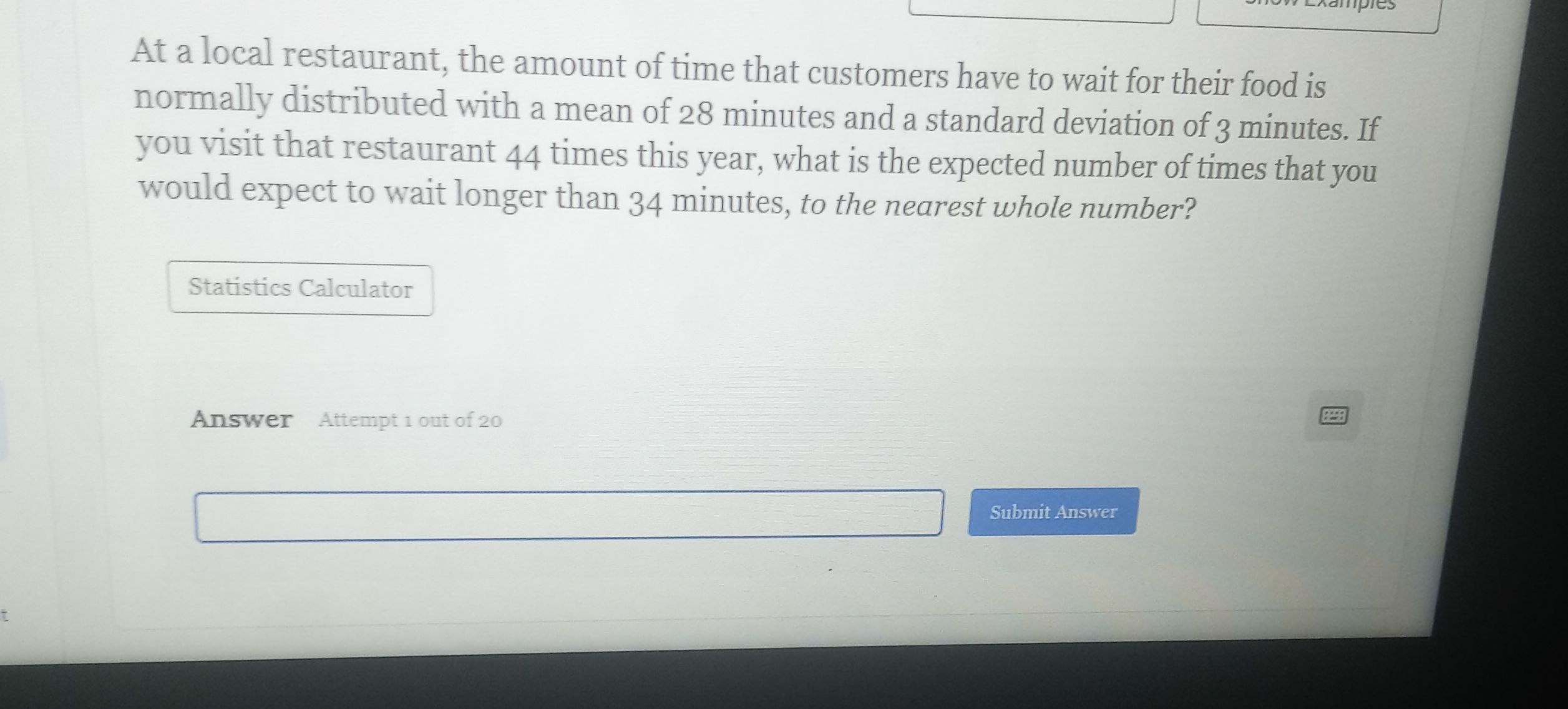
At a local restaurant, the amount of time that customers have to wait for their food is normally distributed with a mean of 28 minutes and a standard deviation of 3 minutes. If you visit that restaurant 44 times this year, what is the expected number of times that you would expect to wait longer than 34 minutes, to the nearest whole number?

Question

Bytelearn · Accepted Answer

Identify Parameters: Identify the parameters of the normal distribution. The mean (μ) is 28 minutes, and the standard deviation (σ) is 3 minutes. We want to find the probability of waiting longer than 34 minutes.Calculate Z-Score: Calculate the z-score for 34 minutes. The z-score formula is $ z = \frac{(X - \mu)}{\sigma} $, where X is the value we are comparing to the mean. So, $ z = \frac{(34 - 28)}{3} = \frac{6}{3} = 2 $.Find Probability: Use the z-score to find the probability of waiting longer than 34 minutes. A z-score of 2 corresponds to a probability of about 0.9772 for the area to the left of the z-score in the standard normal distribution table. To find the probability of waiting longer than 34 minutes, we need to find the area to the right of the z-score, which is $ 1 - 0.9772 = 0.0228 $.Calculate Expected Number: Calculate the expected number of times you will wait longer than 34 minutes out of 44 visits. To find the expected number of times, multiply the probability by the number of visits. $ 0.0228 \times 44 \approx 1.0032 $.Round Result: Round the result to the nearest whole number. Since we can't visit a fraction of a time, we round 1.0032 to the nearest whole number, which is 1.

Full solution

More problems from Find probabilities using the binomial distribution

Related topics