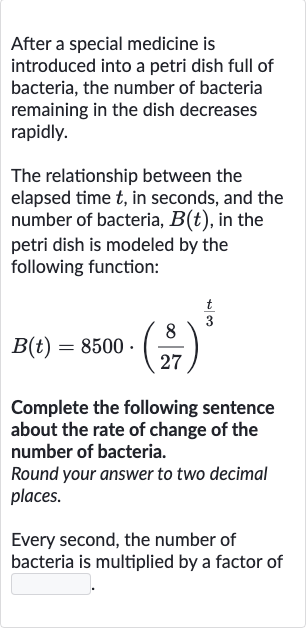
After a special medicine is introduced into a petri dish full of bacteria, the number of bacteria remaining in the dish decreases rapidly. $\newline$ The relationship between the elapsed time $t$ , in seconds, and the number of bacteria, $B(t)$ , in the petri dish is modeled by the following function: $\newline$ $B(t)=8500 \cdot\left(\frac{8}{27}\right)^{\frac{t}{3}}$ $\newline$ Complete the following sentence about the rate of change of the number of bacteria. $\newline$ Round your answer to two decimal places. $\newline$ Every second, the number of bacteria is multiplied by a factor of

Full solution

Q. After a special medicine is introduced into a petri dish full of bacteria, the number of bacteria remaining in the dish decreases rapidly.

\newline

The relationship between the elapsed time

t

, in seconds, and the number of bacteria,

B(t)

, in the petri dish is modeled by the following function:

\newline

B(t)=8500 \cdot\left(\frac{8}{27}\right)^{\frac{t}{3}}

\newline

Complete the following sentence about the rate of change of the number of bacteria.

\newline

Round your answer to two decimal places.

\newline

Every second, the number of bacteria is multiplied by a factor of

Identify Function and Variable: Identify the given function and the variable of interest. $\newline$ The function $B(t) = 8500 \times \left(\frac{8}{27}\right)^{\frac{t}{3}}$ models the number of bacteria over time.
Determine Exponential Function Base: Determine the base of the exponential function that represents the rate of change per second. $\newline$ The base is $(\frac{8}{27})$ , which is raised to the power of $(\frac{t}{3})$ . To find the rate of change per second, we need to evaluate the base raised to the power of $1$ , since $t$ is measured in seconds.
Calculate Multiplication Factor: Calculate the factor by which the number of bacteria is multiplied every second. The factor is $(\frac{8}{27})^{\frac{1}{3}}$ , because every second $t$ increases by $1$ , and we are interested in the change from $t$ to $t+1$ .
Simplify Expression for Factor: Simplify the expression to find the factor. $\newline$ Factor = $(\frac{8}{27})^{\frac{1}{3}}$ $\newline$ Use a calculator to find the cube root of $\frac{8}{27}$ . $\newline$ Factor $\approx (\frac{2}{3})^{\frac{1}{3}}$ $\newline$ Factor $\approx 0.7937$ (rounded to four decimal places)
Round Factor to Two Decimal Places: Round the factor to two decimal places as instructed. $\newline$ Factor $\approx 0.79$ (rounded to two decimal places)